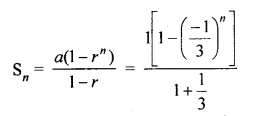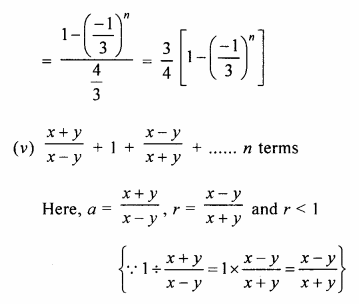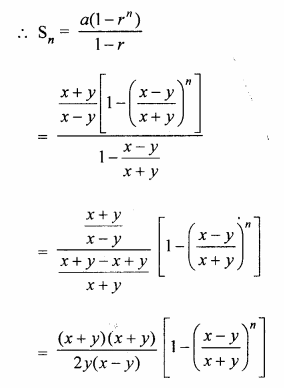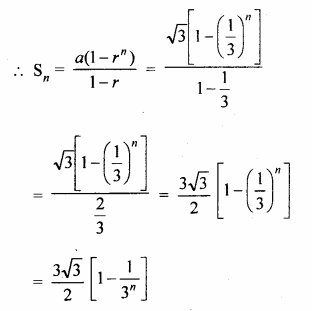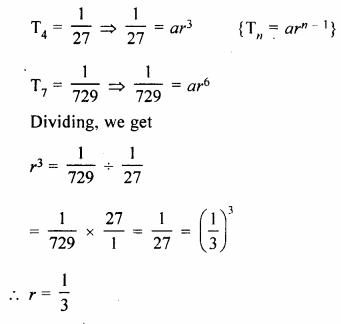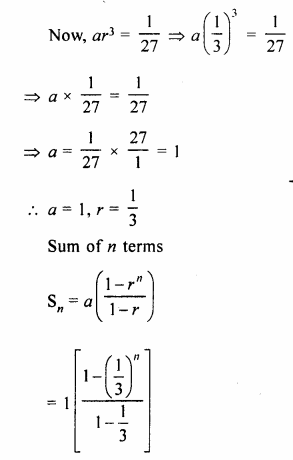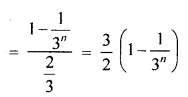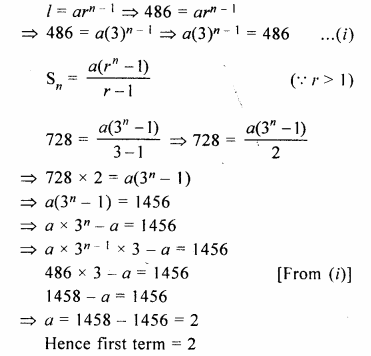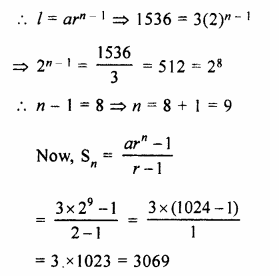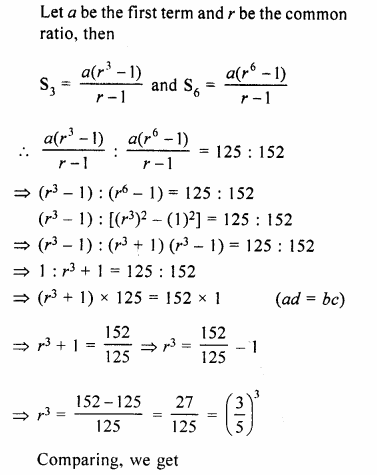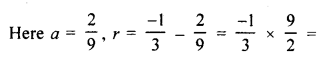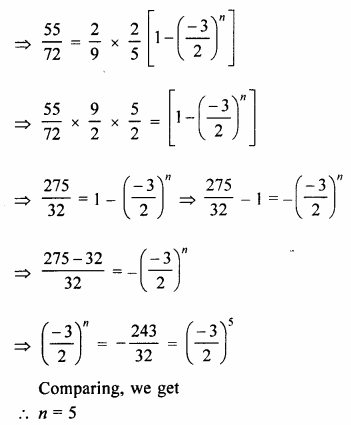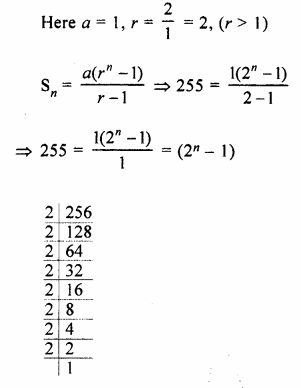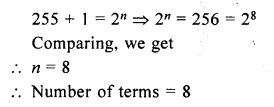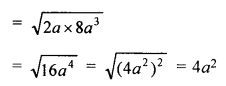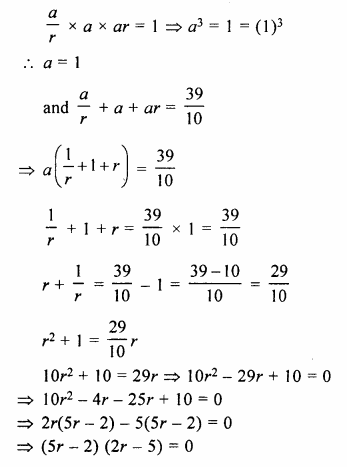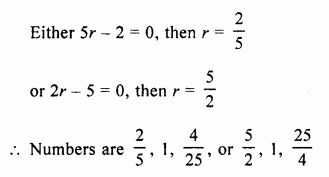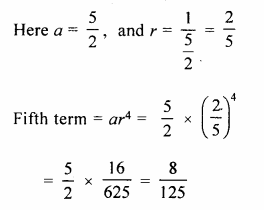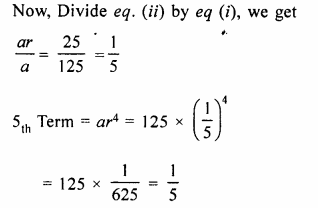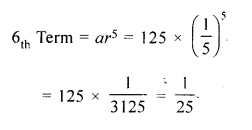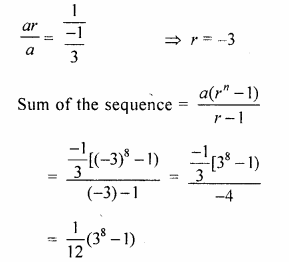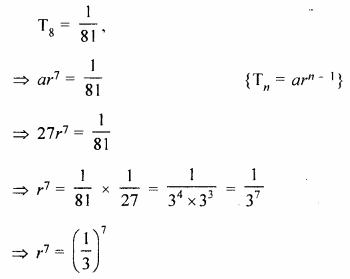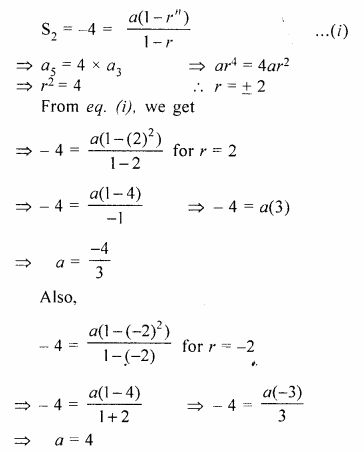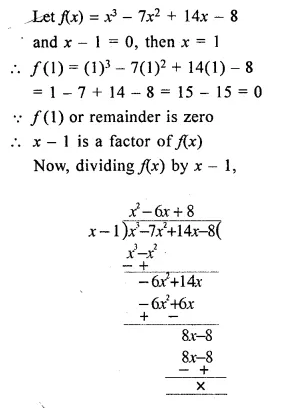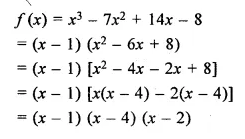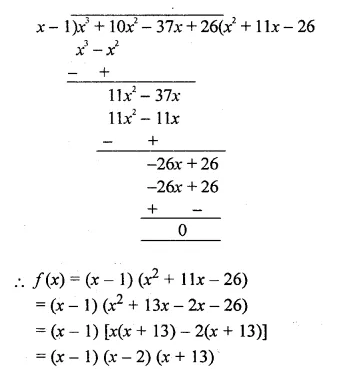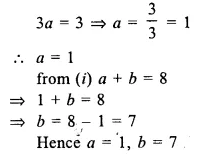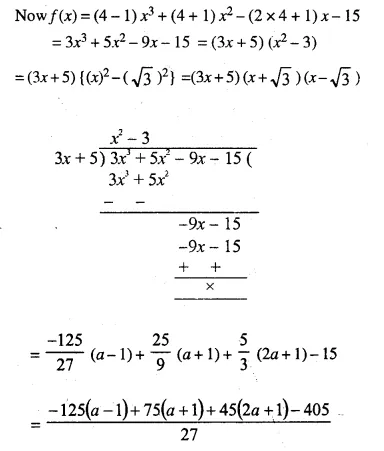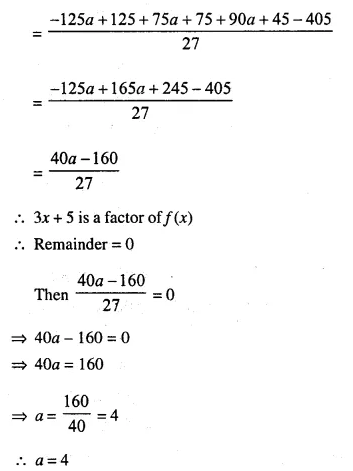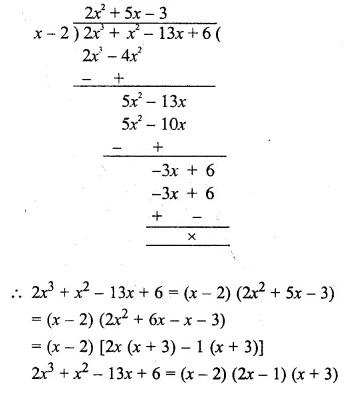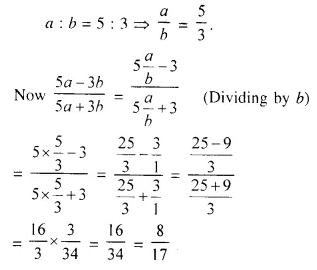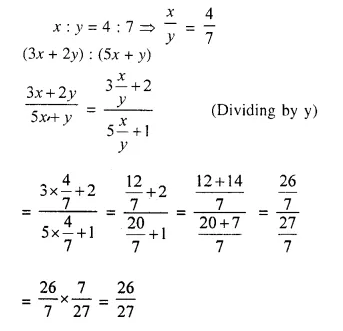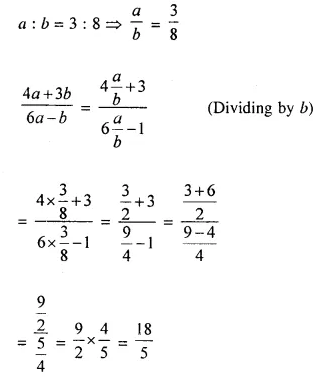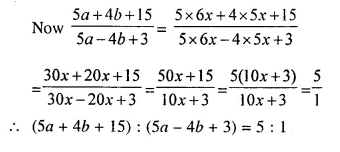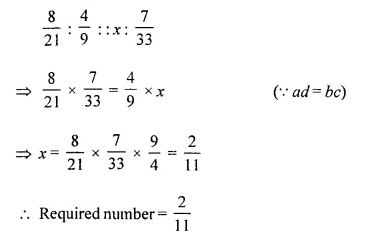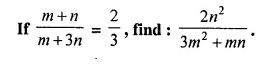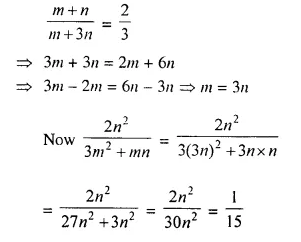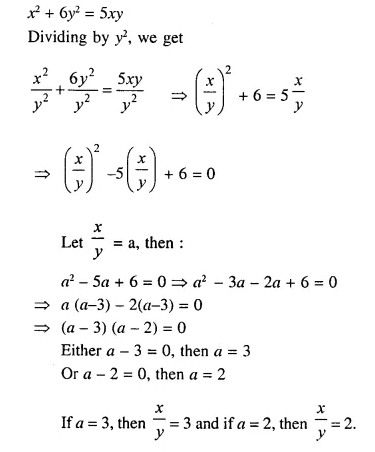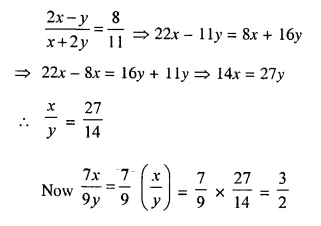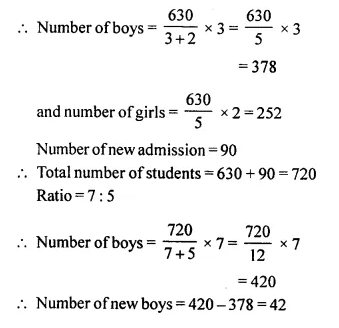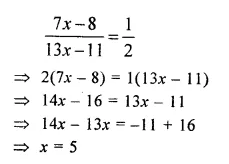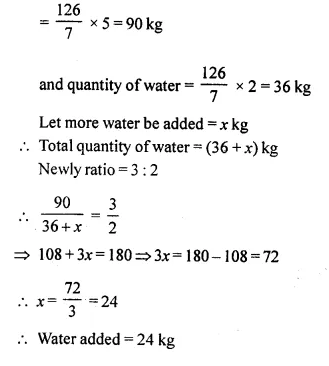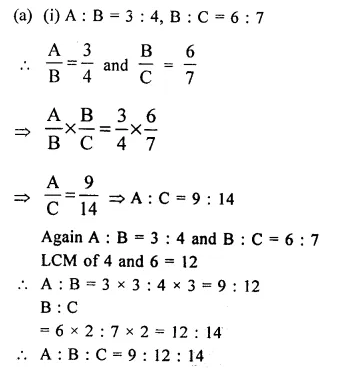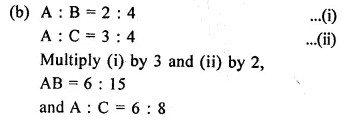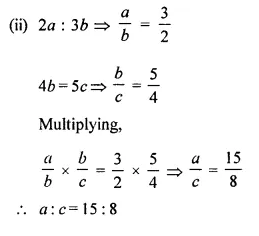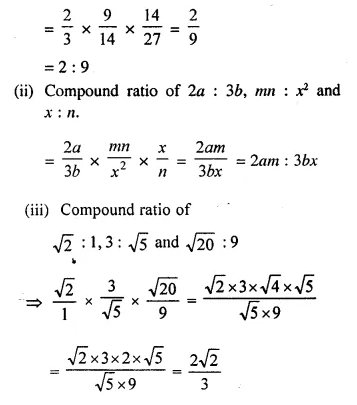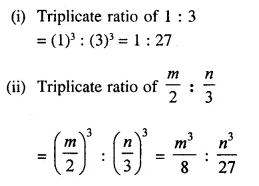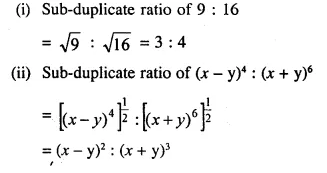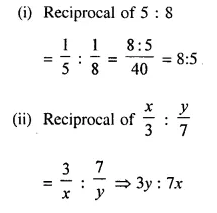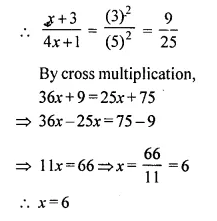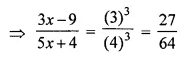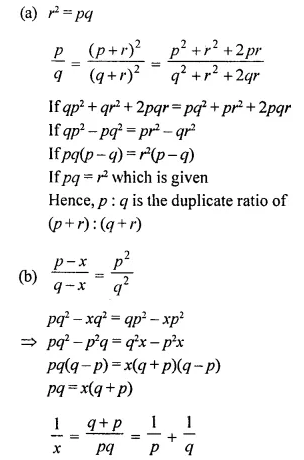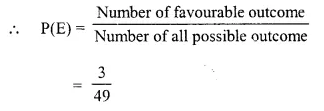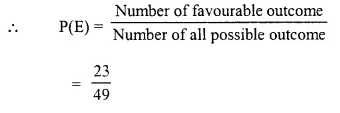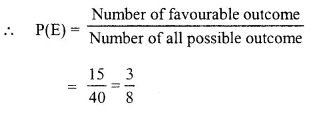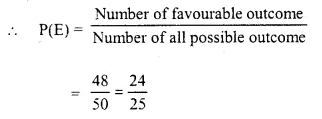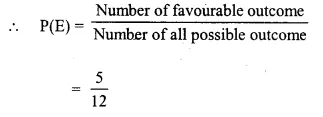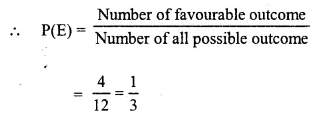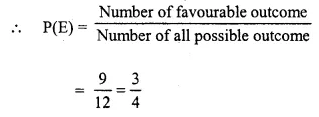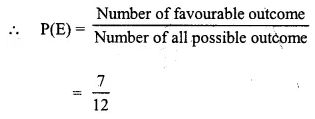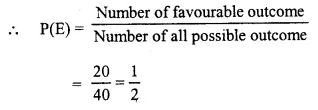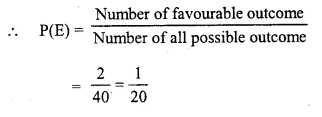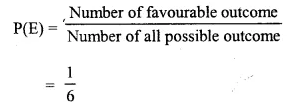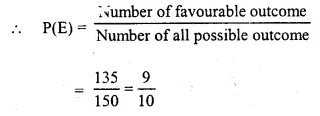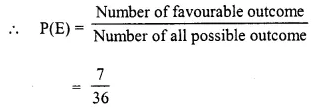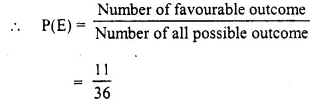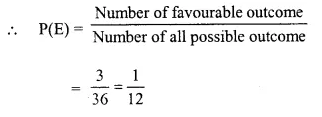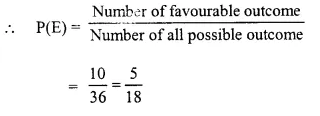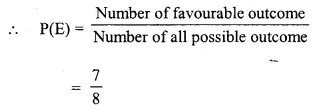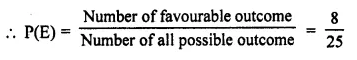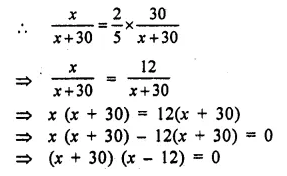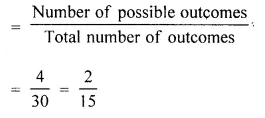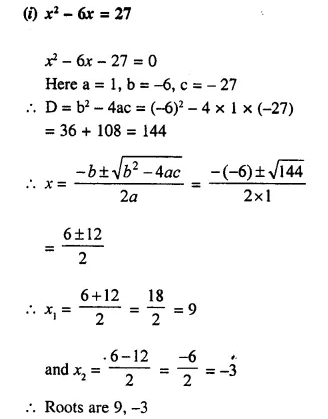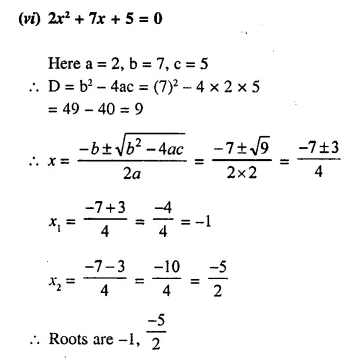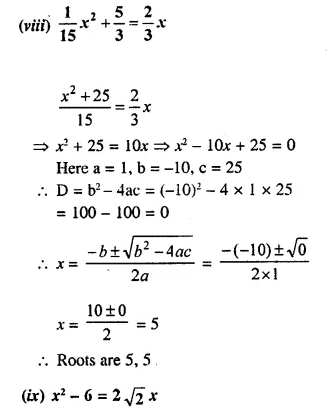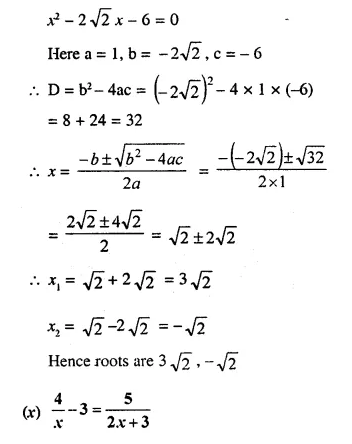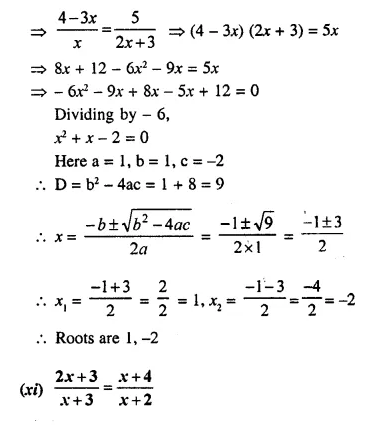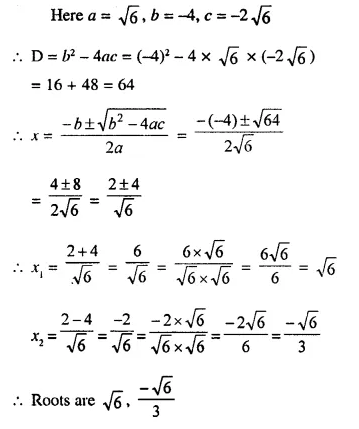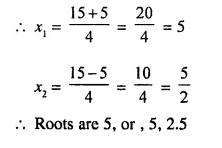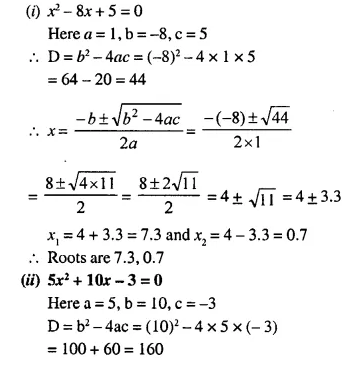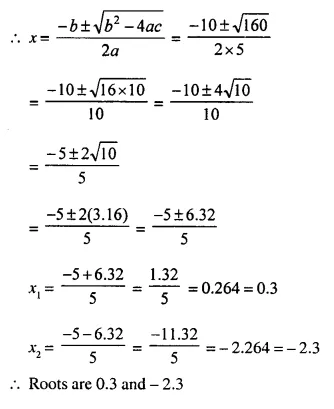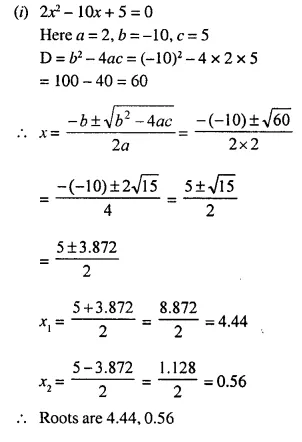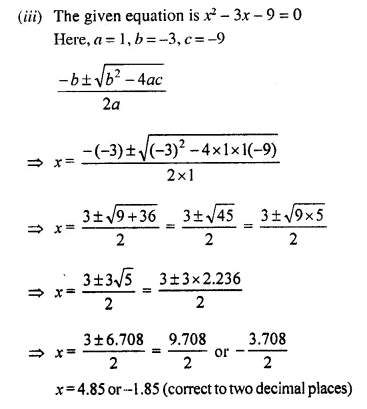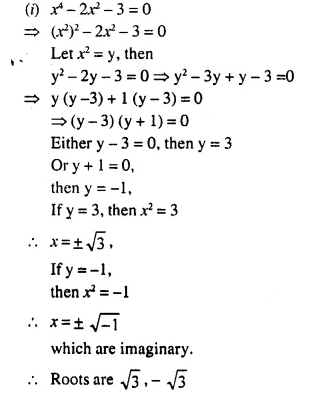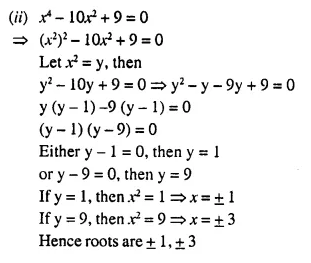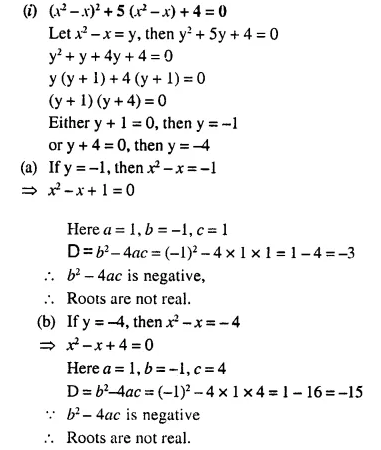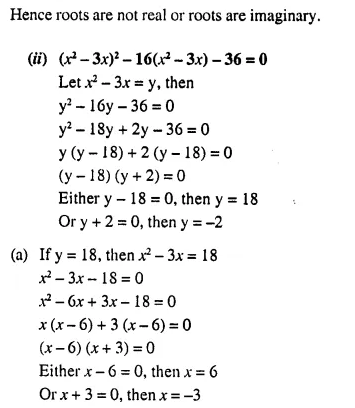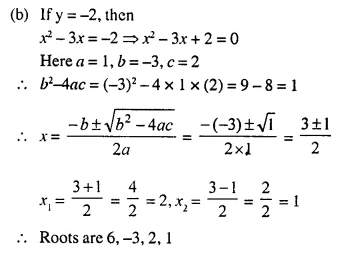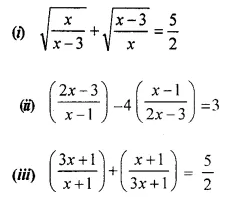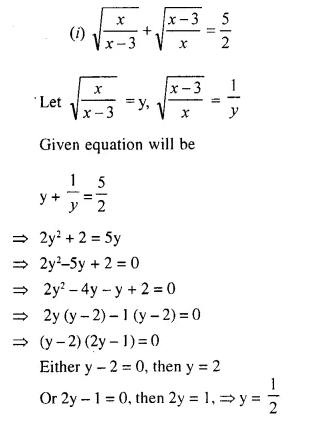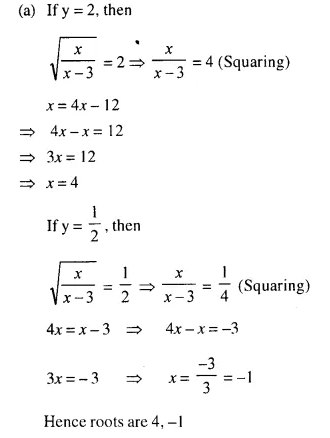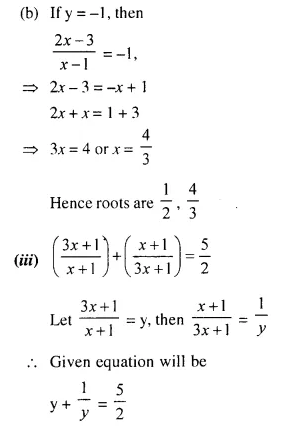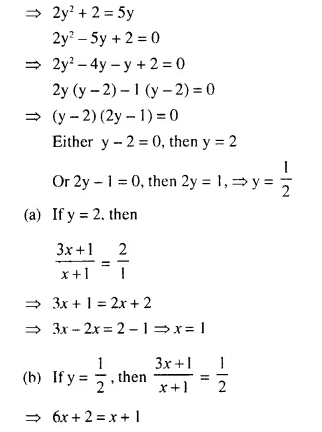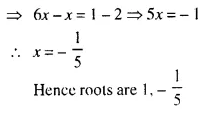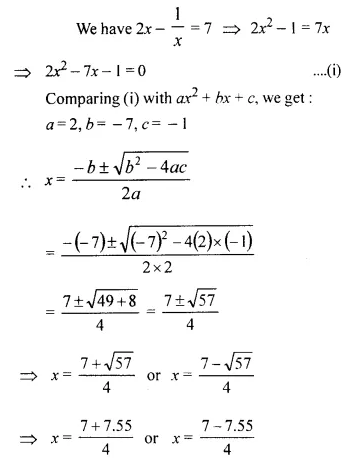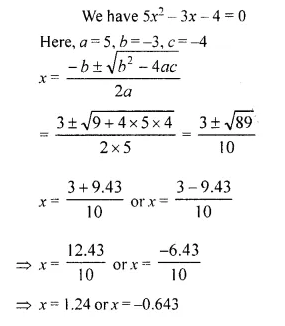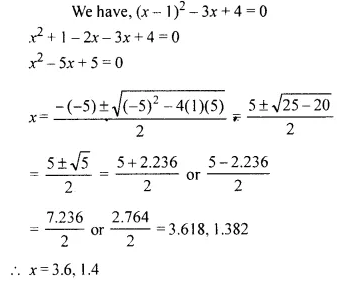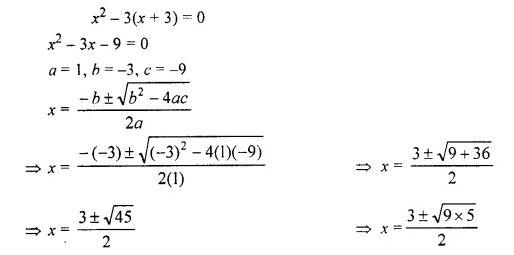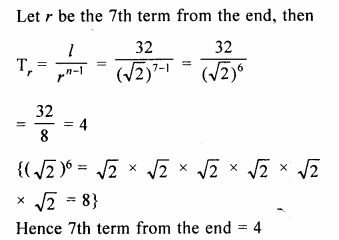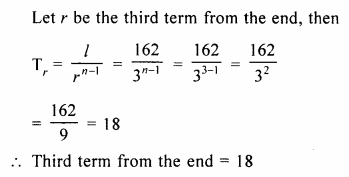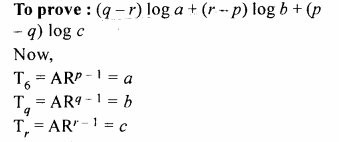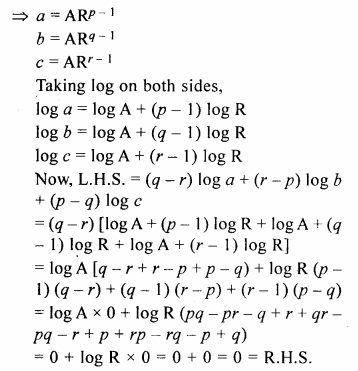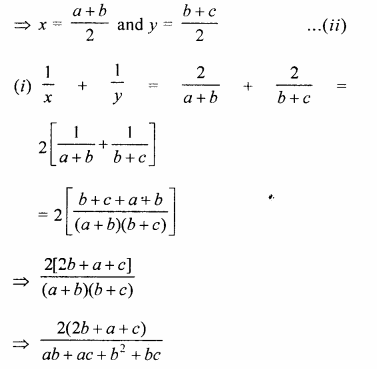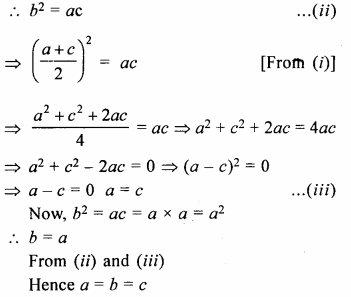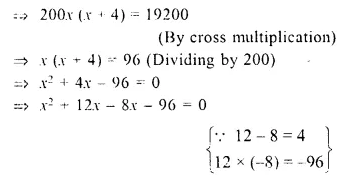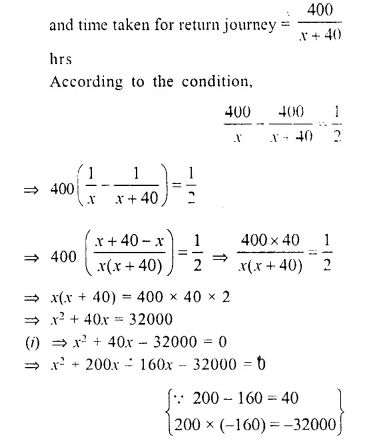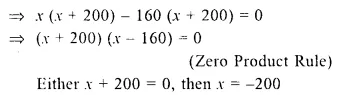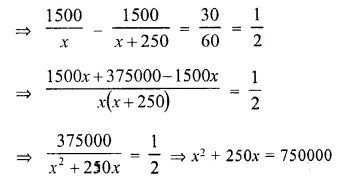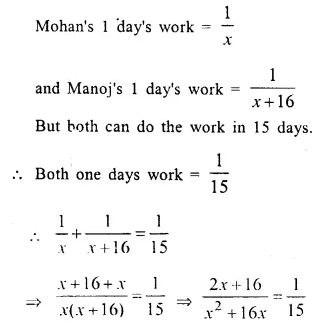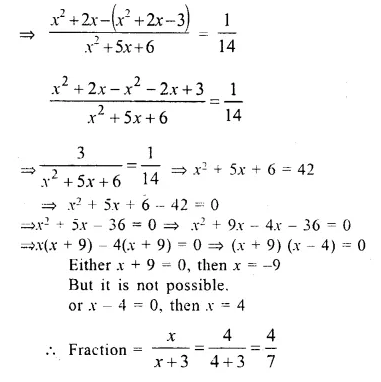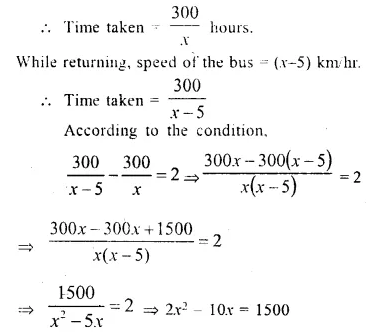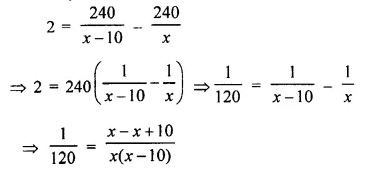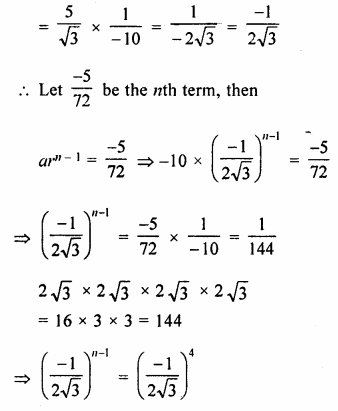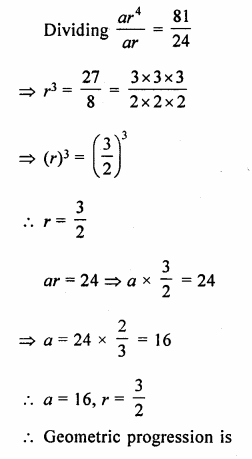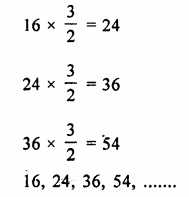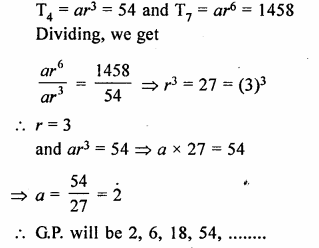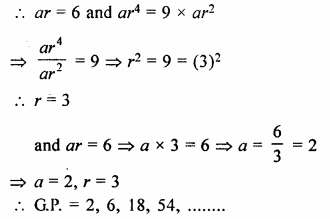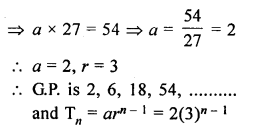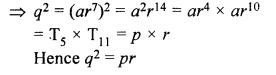Category: ICSE Class 10

Selina Concise Mathematics Class 10 ICSE Solutions Chapter 7 Ratio and Proportion (Including Properties and Uses) Ex 7B

These Solutions are part of Selina Concise Mathematics Class 10 ICSE Solutions. Here we have given Selina Concise Mathematics Class 10 ICSE Solutions Chapter 7 Ratio and Proportion Ex 7B.

Other Exercises

• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 7 Ratio and Proportion Ex 7A
• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 7 Ratio and Proportion Ex 7B
• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 7 Ratio and Proportion Ex 7C
• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 7 Ratio and Proportion Ex 7D

Question 1.
Find the fourth proportional to :
(i) 1.5, 4.5 and 3.5
(ii) 3a, 6a² and 2ab²
Solution:
(i) Let x be the fourth proportional to 1.5, 4.5

Question 2.
Find the third proportional to :
(i) 2\(\frac { 2 }{ 3 }\) and 4
(ii) a – b and a² – b²
Solution:

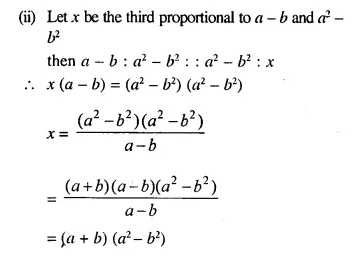
Question 3.
Find the mean proportional between :
(i) 6 + 3√3 and 8 – 4√3
(ii) a – b and a³ – a²b.
Solution:

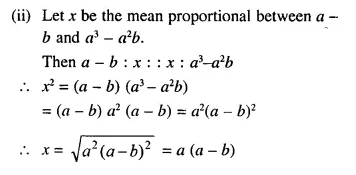
Question 4.
If x + 5 is the mean proportion between x + 2 and x + 9 ; find the value of x.
Solution:
x + 5 is the mean proportion between x + 2 and x + 9
(x + 5)² = (x + 2) (x + 9) {b² = ac}
⇒ x² + 10x + 25 = x² + 11x + 18
⇒ x² + 10x – x² – 11x = 18 – 25
⇒ -x = -7
⇒ x = 7
Hence x = 7

Question 5.
If x², 4 and 9 are in continued proportion, find x.
Solution:
x², 4 and 9 are in continued proportion

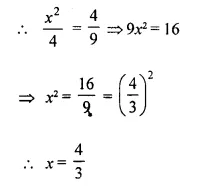
Question 6.
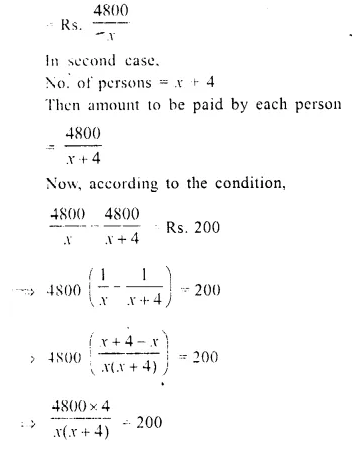
What least number must be added to each of the numbers 6, 15, 20 and 43 to make them proportional. (2005, 2013)
Solution:
Let x to be added to each number then 6 + x, 15 + x, 20 + x and 43 + x are in proportion.

Question 7.

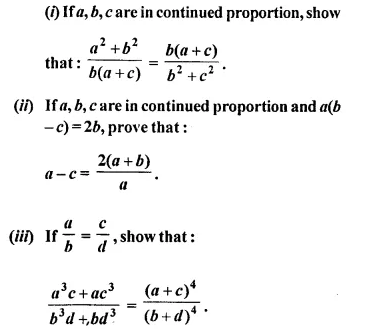
Solution:

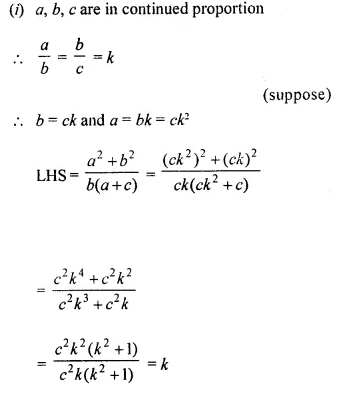
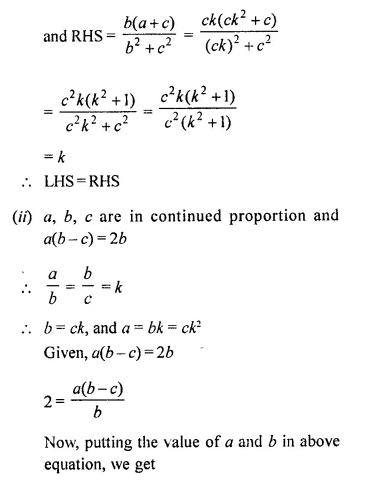
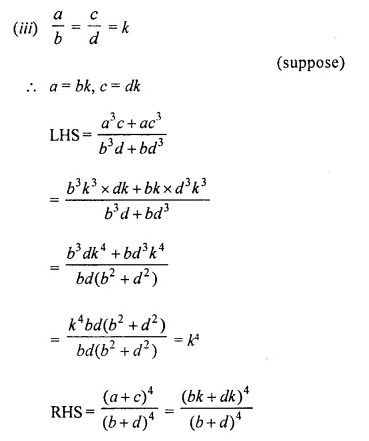
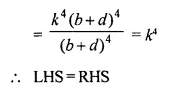
Question 8.
What least number must be subtracted from each of the numbers 7,17 and 47 so that the remainders are in continued pro-portion ?
Solution:
Let x be subtracted from each of the numbers 7, 17 and 47.
Then 7 – x, 17 – x and 47 – x are in continued proportion.
7 – x : 17 – x : : 17 – x : 47 – x
⇒ (7 – x) (47 – x) = (17 – x) (17 – x)
⇒ 329 – 7x – 47x + x² = 289 – 17x – 17x + x²
⇒ -7x – 47x + x² + 17x + 17x – x² = 289 – 329
⇒ -54x + 34x = – 40
⇒ -20x = -40
⇒ x = 2
2 is to be the subtracted

Question 9.
If y is the mean proportional between x and z; show that xy + yz is the mean proportional between x² + y² and y² + z².
Solution:
y is the mean proportional between x and z.
y² = xz
Now, we have to prove that
xy + yz is the mean proportional between x² + y² and y² + z²
i.e., (xy + yz)² = (x² + y²) (y² + z²)
L.H.S. (xy + yz)² = [y(x + z)]² = y² (x + z)² = xz (x + z)²
R.H.S. (x² + y²) (y² + z²) = (x² + xz) (xz + z²) = x (x + z) z (x + z) = xz (x + z)²
L.H.S. = R.H.S.
Hence proved.

Question 10.
If q is the mean proportional between p and r, show that: pqr (p + q + r)³ = (pq + qr + pr)³.
Solution:
q is the mean proportional between p and r,
q² = pr
Now L.H.S. = pqr (p + q + r)³
= qq² (p + q + r)³
= q³ (p + q + r)³
= [q(p + q + r)]³
= (pq + q² + qr)³
= (pq + pr + qr)³
= (pq + qr + pr)³
= R.H.S.

Question 11.
If three quantities are in continued proportion; show that the ratio of the first to the third is the duplicate ratio of the first to the second.
Solution:
Let x, y and z are three quantities which are
in continued proportion
Then x : y : y : z ⇒ y² = zx
Now, we have to prove that
x : z = x² : y² or xy² = zx²
L.H.S. = xy² = x x zx (y² = zx)
= x² z = R.H.S.
Hence Proved.

Question 12.
If y is the mean proportional between x and z, prove that:

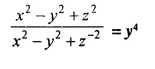
Solution:

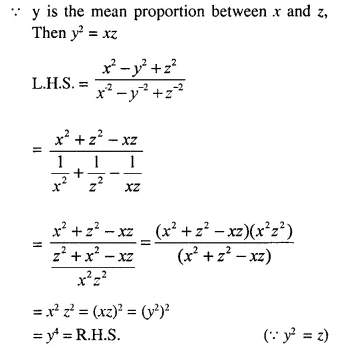
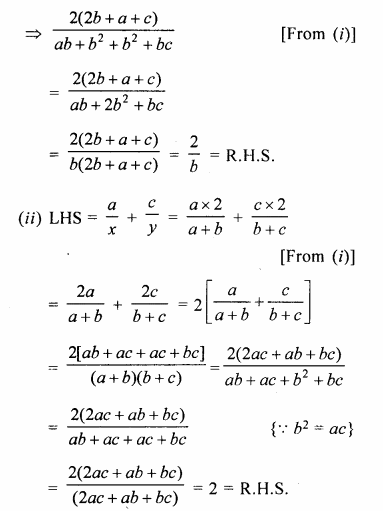
Question 13.
Given four quantities a, b, c and d are in proportion. Show that:
(a – c) b² : (b – d) cd = (a² – b² – ab) : (c² – d² – cd)
Solution:
a, b, c and d are in proportion Then a : b :: c : d

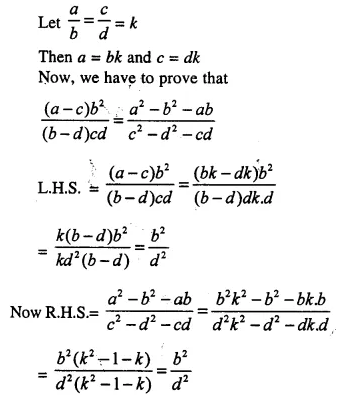
Question 14.
Find two numbers such that the mean proportional between them is 12 and the third proportional to them is 96.
Solution:
Let a and b be the two numbers, whose mean proportional is 12

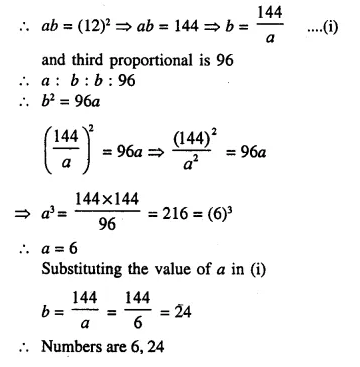
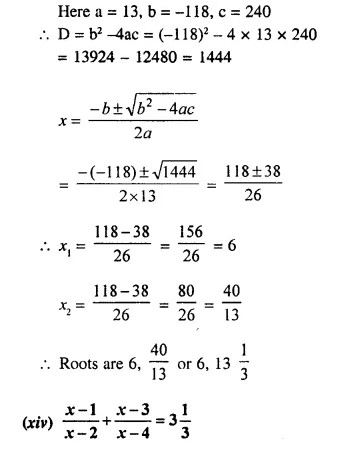
Question 15.

Solution:

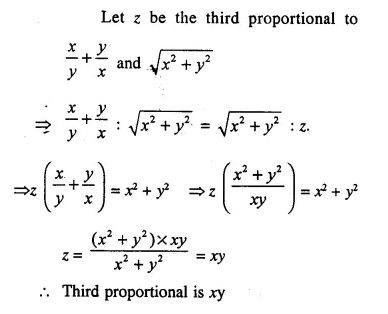
Question 16.
If p : q = r : s ; then show that: mp + nq : q = mr + ns : s.
Solution:

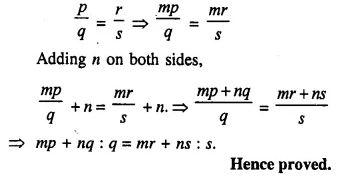
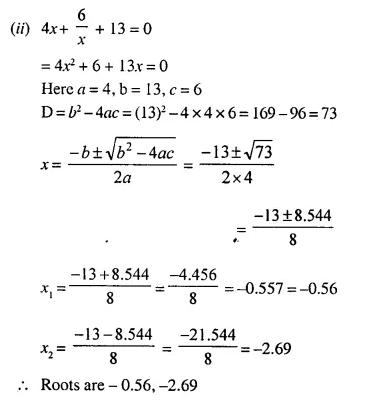
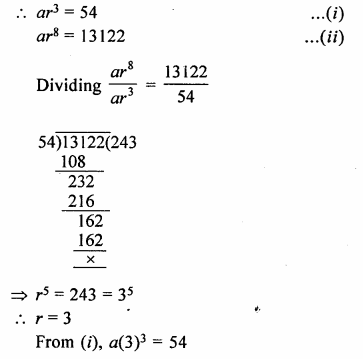
Question 17.

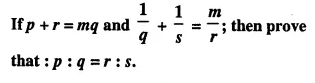
Solution:

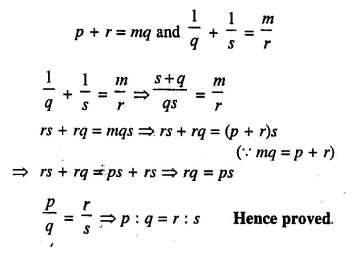
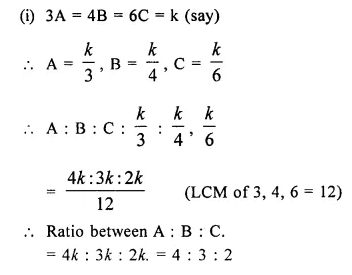
Question 18.

Solution:

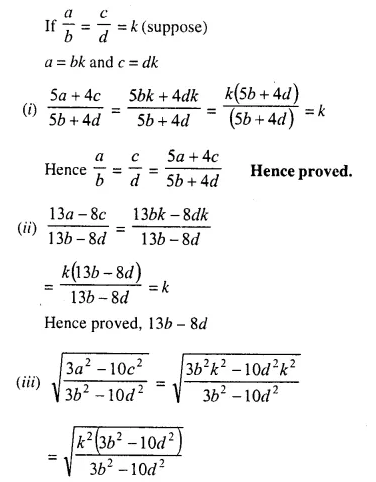
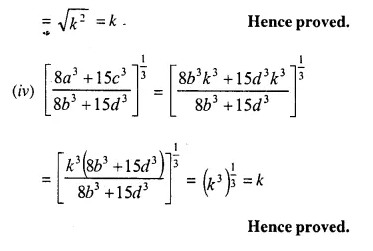
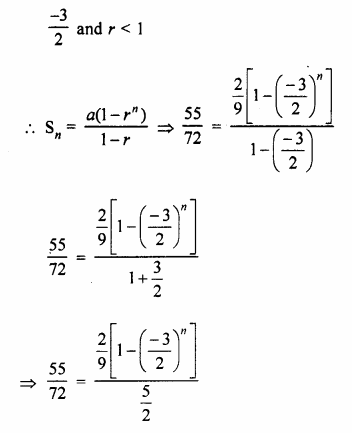
Question 19.

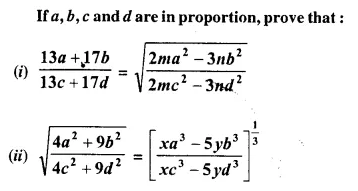
Solution:

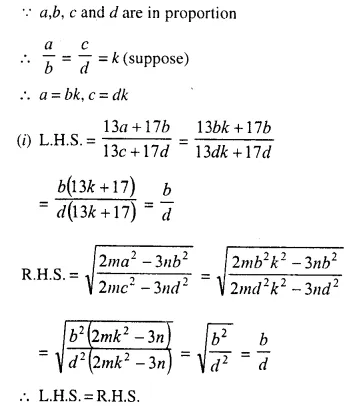
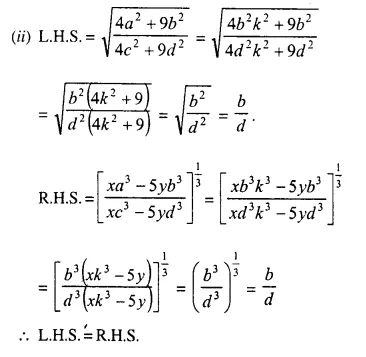
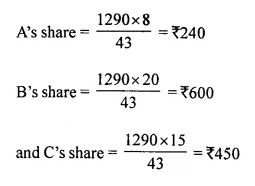
Question 20.

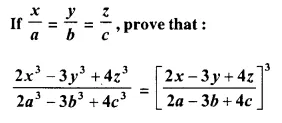
Solution:

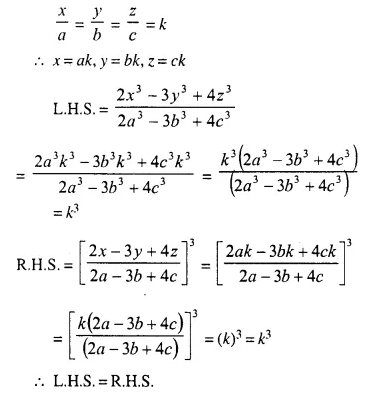
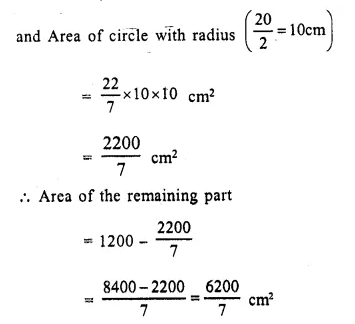
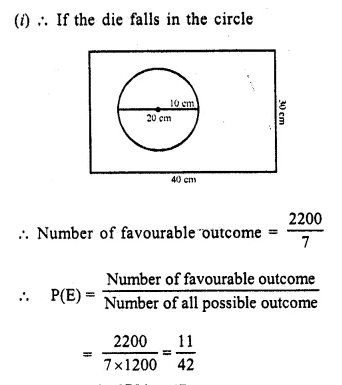
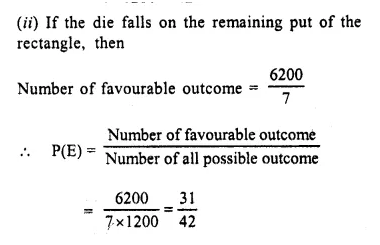
Hope given Selina Concise Mathematics Class 10 ICSE Solutions Chapter 7 Ratio and Proportion Ex 7B are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.

Selina Concise Mathematics Class 10 ICSE Solutions Chapter 22 Heights and Distances Ex 22A

These Solutions are part of Selina Concise Mathematics Class 10 ICSE Solutions. Here we have given Selina Concise Mathematics Class 10 ICSE Solutions Chapter 22 Heights and Distances Ex 22A

Other Exercises

• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 22 Heights and Distances Ex 22A
• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 22 Heights and Distances Ex 22B
• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 22 Heights and Distances Ex 22C

Question 1.
The height of a tree is \(\sqrt { 3 }\) times the length of its shadow. Find the angle of elevation of the sun.
Solution:
Let AB be the tree and BC be its shadow.

∴ θ = 60°
∵ Angle of elevation of the sun = 60°

Question 2.
The angle of elevation of the top of a tower, from a point on the ground and at a distance of 160 m from its foot, is found to be 60°. Find the height of the tower.
Solution:
Let AB be the tower and C is the point which is 160 m away from the foot of the tower,
i.e. CB = 160 m
Let height of the tower be x

Question 3.
A ladder is placed along a wall such that its upper end is resting against a vertical wall. The foot of the ladder is 2.4 m from the wall and the ladder is making an angle of 68“ with the ground. Find the height, upto which the ladder reaches.
Solution:
Let AB be the wall and

AC be the ladder, which is placed against the wall. If foot is 2.4 m away from the wall i.e. CB = 2.4m₁.
Let AB =x m.
In right ∆ ABC,
tan θ = \(\frac { AB }{ BC }\) ⇒ tan 68° = \(\frac { X }{ 2.4 }\)
∴ x = 2.4 x tan 68° = 2.4 x 2.4751
= 5.94 m

Question 4.
Two persons are standing on the opposite sides of a tower. They observe the angles of elevation of the top of the tower to be 30° and 38° respectively. Find the distance between them, if the height of the tower is 50 m.
Solution:

Two persons A and B are standing on the opposite side of the tower TR and height of tower TR = 50 m and angles of elevation with A and B are 30° and 38° respectively. Let AR = x and RB = y
Now in right ∆TAR,

Question 5.
A kite is attached to a string. Find the length of the string, when the height of the kite is 60 m. and the string makes an angle 30° with the ground.
Solution:
Let KT be the height of kite and PK is the string which makes an angle of 30° with the ground.
∴ KT = 60 m
Let KP = xm.
Now in right ∆PKT,

Question 6.
A boy 1.6m tall, is 20 m away from a tower and observes the angle of elevation of the top of the tower to be (i) 45° (ii) 60°. Find the height of the tower in each case.
Solution:
(i) Let AB be the tower and MN be the boy who is 20m away from the foot of the tower.
Let AB = x and angle of elevation = 45°

Question 7.
The upper part of a tree, broken over by the wind, makes an angle of 45° with the ground; and the distance from the root to the point where the top of the tree touches the ground, is 15m. What was the height of the tree before it was broken ?
Solution:
Let AB be the tree which was broken at the point C which makes an angle of elevation of 45°, with the ground at a distance of 15m.
BD = 15m
AC = CD

Question 8.
The angle of elevation of the top of an unfinished tower at a point distance 80 m from its base is 30°. How much higher must the tower be raised so that its angle of elevation at the same point may be 60° ?
Solution:
Distance of a point from the tower = 80 m
Angle of elevation = 30°
In second case the elevation of lower = 60°
In first case,

Question 9.
At a particular time, when the sun’s altitude is 30°, the length of the shadow of’C vertical tower is 45 m. Calculate :
(i) height of the tower.
(ii) the length of the shadow of the same tower, when the sun’s altitude is (a) 45° (b) 60°.
Solution:
Shadow of the tower = 45 m and angle of elevation = 30°
Let AB be the lower and BC is its shadow.
∴ CB = 45 m.
Now in right ∆ABC,

(ii) In second case,
(a) Angle of elevation = 45°
and height of tower = 25.98 m or 15\(\sqrt { 3 }\) m

(b) Angle of elevation = 60°
and height of tower = 25.98 m or 15\(\sqrt { 3 }\) m.
Let shadow of the tower DB = xm

Question 10.
Two vertical poles are on either side of a road. A 30 m long ladder is placed between the two poles. When the ladder rests against one pole, it makes angle 32°24′ with the pole and when it is turned to rest against another pole, it makes angle 32°24′ with the road. Calculate the width of the road.
Solution:
Two poles AB and CD which are at the either end of a road BD. A ladder 30 m long subtends an angle of 32° 24′ with the first pole AB and 32°24′ with the road when it is turned to rest against the second pole CD.
Now in right ∆ABE.

Question 11.
Two climbers are at points A and Bona vertical cliff face. To an observer C, 40 m from the foot of the cliff on the level ground, A is at an elevation of 48° and B of 57°. What is the distance between the climbers ?
Solution:
A and B are two climbers on the cliff and ob-server is at C, 40 m from the foot of the cliff while the angles of elevations of each climber is 48° and 57° respectively.
In right ∆ACD,

Question 12.
A man stands 9m away from a flag-pole. He observes that angle of elevation of the top of the pole is 28° and the angle of depression of the bottom of the pole is 13°. Calculate the height of the pole.
Solution:
Let PL is the pole and MN is the man The angle of elevation of the top of the pole = 28°
arid the angle of depression of the bottom of the pole =13°
Man is 9 m away from the pole,
i.e. MQ = 9 m
Now in right ∆PMQ,

Question 13.
From the top of a cliff 92 m high, the angle of depression of a buoy is 20°. Calculate to the nearest metre the distance of the buoy from the foot of the cliff.
Solution:
Let CD be the cliff and CD = 92m, B is the buoy,
then from C ,
the angle of depression is 20°

Hope given Selina Concise Mathematics Class 10 ICSE Solutions Chapter 22 Heights and Distances Ex 22A are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.

Selina Concise Mathematics Class 10 ICSE Solutions Chapter 11 Geometric Progression Ex 11D

These Solutions are part of Selina Concise Mathematics Class 10 ICSE Solutions. Here we have given Selina Concise Mathematics Class 10 ICSE Solutions Chapter 11 Geometric Progression Ex 11D

Other Exercises

• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 11 Geometric Progression Ex 11A
• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 11 Geometric Progression Ex 11B
• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 11 Geometric Progression Ex 11C
• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 11 Geometric Progression Ex 11D
• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 11 Geometric Progression Additional Questions

Question 1.
Find the sum of G.P. :
(i) 1 + 3 + 9 + 27 +….to 12 terms.
(ii) 0.3 + 0.03 + 0.003 + 0.0003 +….to 8 terms.
(iii) \(1-\frac { 1 }{ 2 } +\frac { 1 }{ 4 } -\frac { 1 }{ 8 } ….to\quad 9\quad terms\)
(iv) \(1-\frac { 1 }{ 3 } +\frac { 1 }{ { 3 }^{ 2 } } -\frac { 1 }{ { 3 }^{ 3 } } ….to\quad n\quad terms \)
(v) \(\frac { x+y }{ x-y } +1+\frac { x-y }{ x+y } +….upto\quad n\quad terms\)
(vi) \(\sqrt { 3 } +\frac { 1 }{ \sqrt { 3 } } +\frac { 1 }{ 3\sqrt { 3 } } +….to\quad n\quad terms\)
Solution:
(i) 1 + 3 + 9 + 27 +….to 12 terms.
Here a = 1, r = 3 and n = 12

Question 2.
How many terms of the geometric progression 1 + 4 + 16 + 64 +…. must be added to get sum equal to 5461 ?
Solution:
S_n = 5461 and G.P. is
1 + 4 + 16 + 64 +…..
Here, a = 1, r = 4 (r > 1)

Question 3.
The first term of a G.P. is 27 and its 8th term is \(\\ \frac { 1 }{ 81 } \). Find the sum of its first 10 terms.
Solution:
First term of a G.P (a) = 27
T₈ = \(\\ \frac { 1 }{ 81 } \), n = 10
a = 27

Question 4.
A boy spends Rs 10 on first day, Rs 20 on second day, Rs 40 on third day and so on. Find how much, in all, will he spend in 12 days?
Solution:
A boy spends Rs 10 on first day,
Rs 20 on second day
Rs 40 on third day and so on
G.P. is 10 + 20 + 40 +…. 12 terms
Here a = 10, r = 2 and n = 12 (r > 1)

Question 5.
The 4th and the 7th terms of a G.P. are \(\\ \frac { 1 }{ 27 } \) and \(\\ \frac { 1 }{ 729 } \) respectively. Find the sum of n terms of this G.P.
Solution:
In a G.P.
T₄ = \(\\ \frac { 1 }{ 27 } \)

Question 6.
A geometric progression has common ratio = 3 and last term = 486. If the sum of its terms is 728 ; find its first term.
Solution:
In a G.P.
Common ratio (r) = 3
Last term (l) = 486
Sum of its terms (S_n) = 728
Let a be the first term, then

Question 7.
Find the sum of G.P. : 3, 6, 12, …… 1536.
Solution:
G.P. is 3, 6, 12,….1536
Here a = 3, r = \(\\ \frac { 6 }{ 3 } \) = 2

Question 8.
How many terms of the series 2 + 6 + 18 +…. must be taken to make the sum equal to 728 ?
Solution:
G.P. is 2 + 6 + 18 +….
Here a = 2, r = \(\\ \frac { 6 }{ 2 } \) = 3, S_n = 728

Question 9.
In a G.P., the ratio between the sum of first three terms and that of the first six terms is 125 : 152. Find its common ratio.
Solution:
In a G.P.
Sum of first 3 terms : Sum of 6 terms = 125 : 152

Question 10.
Find how many terms of G.P.\(\frac { 2 }{ 9 } -\frac { 1 }{ 3 } +\frac { 1 }{ 2 } \)… must be added to get the sum equal to \(\\ \frac { 55 }{ 72 } \) ?
Solution:
\(\frac { 2 }{ 9 } -\frac { 1 }{ 3 } +\frac { 1 }{ 2 } \)…
Let n terms be added
Now,S_n = \(\\ \frac { 55 }{ 72 } \)

Question 11.
If the sum of 1 + 2 + 2² +…..+ 2^{n – 1} is 255, find the value of n.
Solution:
1 + 2 + 2² +…..+ 2^{n – 1} = 255

Question 12.
Find the geometric mean between :
(i) \(\\ \frac { 4 }{ 9 } \) and \(\\ \frac { 9 }{ 4 } \)
(ii) 14 and \(\\ \frac { 7 }{ 32 } \)
(iii) 2a and 8a³
Solution:
(i) G.M between \(\\ \frac { 4 }{ 9 } \) and \(\\ \frac { 9 }{ 4 } \)

Question 13.
The sum of three numbers in G.P. is \(\\ \frac { 39 }{ 10 } \) and their product is 1. Find the numbers.
Solution:
Sum of three numbers in G.P. = \(\\ \frac { 39 }{ 10 } \)
and their product = 1
Let number be \(\\ \frac { a }{ r } \), a, ar, then

Question 14.
The first term of a G.P. is – 3 and the square of the second term is equal to its 4th term. Find its 7th term.
Solution:
In G.P.
T1 = – 3

Question 15.
Find the 5th term of the G.P. \(\\ \frac { 5 }{ 2 } \), 1,…..
Solution:
Given G.P is \(\\ \frac { 5 }{ 2 } \), 1,…..

Question 16.
The first two terms of a G.P. are 125 and 25 respectively. Find the 5th and the 6th terms of the G.P.
Solution:
Given, First term = a = 125….(i)
and Second term = ar = 25…..(ii)
Now, Divide eq. (ii) by eq (i), we get

Question 17.
Find the sum of the sequence \(– \frac { 1 }{ 3 } \), 1, – 3, 9,….upto 8 terms.
Solution:
Here, First Term, a = \(– \frac { 1 }{ 3 } \)…(i)
and Second Term, ar = 1 …(ii)
Dividing eq. (i) by eq. (ii), we get

Question 18.
The first term of a G.P. in 27. If the 8th term be \(\\ \frac { 1 }{ 81 } \), what will be the sum of 10 terms ?
Solution:
Given, First term (a) = 27, n = 10

Question 19.
Find a G.P. for which the sum of first two terms is – 4 and the fifth term is 4 times the third term.
Solution:
Let a be the first term and r be the common ratio of the G.P.
According to the given conditions,

Hope given Selina Concise Mathematics Class 10 ICSE Solutions Chapter 11 Geometric Progression Ex 11D are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.

Selina Concise Mathematics Class 10 ICSE Solutions Chapter 8 Remainder and Factor Theorems Ex 8C

These Solutions are part of Selina Concise Mathematics Class 10 ICSE Solutions. Here we have given Selina Concise Mathematics Class 10 ICSE Solutions Chapter 8 Remainder and Factor Theorems Ex 8C.

Other Exercises

• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 8 Remainder and Factor Theorems Ex 8A
• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 8 Remainder and Factor Theorems Ex 8B
• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 8 Remainder and Factor Theorems Ex 8C

Question 1.
Show that (x – 1) is a factor of x³ – 7x² + 14x – 8. Hence, completely factorise the given expression.
Solution:

Question 2.
Using Remainder Theorem, factorise : x³ + 10x² – 37x + 26 completely. (2014)
Solution:
f(x) = x³ + 10x² – 37x + 26
f(1) = (1)³ + 10(1)² – 37(1) + 26 = 1 + 10 – 37 + 26 = 0
x = 1
x – 1 is factor of f(x)

Question 3.
When x³ + 3x² – mx + 4 is divided by x – 2, the remainder is m + 3. Find the value of m.
Solution:
Let f(x) = x³ + 3x² – mx + 4
and x – 2 = 0 then x = 2
f(2) = (2)³ + 3(2)² – m(2) + 4 = 8 + 12 – 2m + 4 = 24 – 2m
Remainder = 24 – 2m
But, remainder is given m + 3
m + 3 = 24 – 2m
⇒ m + 2m = 24 – 3
⇒ 3m = 21
⇒ m = 7
Hence m = 7

Question 4.
What should be subtracted from 3x³ – 8x² + 4x – 3, so that the resulting expression has x + 2 as a factor ?
Solution:
The number to be subtracted = Remainder obtained by dividing 3x³ – 8x² + 4x – 3 by x + 2
Let f(x) = 3x³ – 8x² + 4x – 3
and x + 2 = 0, then x = – 2
Remainder = f(-2) = 3 (-2)³ – 8 (-2)² + 4 (-2) – 3 = -24 – 32 – 8 – 3 = -67
Hence the number to be subtracted = – 67

Question 5.
If (x + 1) and (x – 2) are factors of x³ + (a + 1) x² – (b – 2) x – 6, find the values of a and 6. And then, factorise the given expression completely.
Solution:

Question 6.
If x – 2 is a factor of x² – ax + b and a + b = 1, find the values of a and b.
Solution:
(x – 2) is a factor of x² + ax + b
Let x – 2 = 0 ⇒ x = 2
Now x² + ax + b = (2)² + a x 2 + b = 4 + 2a + b = 2a + b + 4
x – 2 is the factor Remainder = 0 or 2a + b + 4 = 0
⇒ 2a + b = -4 …(i)
But a + b = 1 (given) …(ii)
Subtracting, we get : a = -5
Substituting the value of a in (ii)
-5 + b = 1 ⇒ b = 1 + 5 ⇒ b = 6
Hence a = -5, b = 6

Question 7.
Factorise x³ + 6x² + 11x + 6 completely using factor theorem.
Solution:

Question 8.
Find the value of ‘m’ if mx³ + 2x² – 3 and x² – mx + 4 leave the same remainder when each is divided by x – 2.
Solution:
Let f(x) = mx³ + 2x² – 3
g (x) = x² – mx + 4
Let x – 2 = 0, then x = 2
f(2) = m (2)³ + 2 (2)² – 3 = 8m + 8 – 3 = 8m + 5
g(2) = (2)² – mx² + 4 = 4 – 2m + 4 = 8 – 2m
In both cases the remainder is same
8m + 5 = 8 – 2m
⇒ 8m + 2m = 8 – 5
⇒ 10m = 3
⇒ m = \(\frac { 3 }{ 10 }\)

Question 9.
The polynomial px³ + 4x² – 3x + q is completely divisible by x² – 1; find the values of p and q. Also, for these values of p and q, factorize the given polynomial completely.
Solution:

Question 10.
Find the number which should be added to x² + x + 3 so that the resulting polynomial is completely divisible by (x + 3).
Solution:
Let k be added to f(x), then f(x) = x² + x + 3 + k
Let x + 3 = 0, then x = -3
f(-3) = (-3)2 + (-3) + 3 + k = 9 – 3 + 3 + k = 9 + k
f(x) is divisible by x + 3, then remainder will be 0.
9 + k = 0 ⇒ k = -9
-9 should be added

Question 11.
When the polynomial x³ + 2x² – 5ax – 7 is divided by (x – 1), the remainder is A and when the polynomial x³ + ax² – 12x + 16 is divided by (x + 2), the remainder is B. Find the value of ‘a’ if 2A + B = 0.
Solution:
Let f(x) = x³ + 2x² – 5ax – 1
and let x – 1 = 0, then x = 1
f(1) = (1)³ + 2(1)² – 5a x 1 – 7 = 1 + 2 – 5a – 7 = -5a – 4
-5a – 4 = A ….(i)
Let g (x) = x³ + ax² +12x + 16
and let x + 2 = 0, then x = -2
g (-2) = (-2)³ + a (-2)² – 12 (-2) + 16 = -8 + 4a + 24 + 16 = 32 + 4a
32 + 4a = B ….(ii)
2A + B = 0
2 (-5a – 4) + 32 + 4a = 0
⇒ -10a – 8 + 32 + 4a = 0
⇒ -6a + 24 = 0
⇒ 6a = 24
⇒ a = 4
a = 4

Question 12.
(3x + 5) is a factor of the polynomial (a – 1) x³ + (a + 1) x² – (2a + 1) x – 15. Find the value of ‘a’. For this value of ‘a’, factorise the given polynomial completely.
Solution:
Let f(x) = (a – 1) x³ + (a + 1) x² – (2a + 1) x – 15

Question 13.
When divided by x – 3 the polynomials x³ – px² + x + 6 and 2x³ – x² – (p + 3) x – 6 leave the same remainder. Find the value of ‘p.’
Solution:
When (x – 3) divides x³ – px² + x + 6,
then Remainder = p(3) = (3)³ – p(3)² + (3) + 6 = 27 – 9p + 9 = 36 – 9p
When (x – 3) divides 2x³ – x² – (p + 3) x – 6,
then Remainder = p(3) = 2(3)³ – (3)² – (p + 3) (3) – 6
= 54 – 9 – 3p – 9 – 6 = 30 – 3p
A.T.Q. both remainders are equal
⇒ 36 – 9p = 30 – 3p
⇒ 36 – 30 = -3p + 9p
⇒ 6 = 6p
⇒ p = 1

Question 14.
Use the Remainder Theorem to factorise the following expression : 2x³ + x² – 13x + 6
Solution:
(a) By hit and trial, putting x = 2, we have
2 (8) + 4 – 26 + 6 = 0
⇒ (x – 2) is the factor of 2x³ + x² – 13x + 6

Question 15.
Using remainder theorem, find the value of k if on dividing 2x³ + 3x² – kx + 5 by x – 2, leaves a remainder 7. (2016)
Solution:
Let f(x) = 2x³ + 3x² – kx + 5 By the remainder theorem,
f(2) = 7
⇒ 2(2)³ + 3(2)² – k(2) + 5 = 7
⇒ 2(8) + 3(4) – k(2) + 5 = 7
⇒ 16 + 12 – 2k + 5 = 7
⇒ 2k = 16 + 12 + 5 – 7
⇒ 2k = 26
⇒ k = 13
The value of k is 13.

Hope given Selina Concise Mathematics Class 10 ICSE Solutions Chapter 8 Remainder and Factor Theorems Ex 8C are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.

Selina Concise Mathematics Class 10 ICSE Solutions Chapter 7 Ratio and Proportion (Including Properties and Uses) Ex 7A

These Solutions are part of Selina Concise Mathematics Class 10 ICSE Solutions. Here we have given Selina Concise Mathematics Class 10 ICSE Solutions Chapter 7 Ratio and Proportion Ex 7A.

Other Exercises

• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 7 Ratio and Proportion Ex 7A
• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 7 Ratio and Proportion Ex 7B
• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 7 Ratio and Proportion Ex 7C
• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 7 Ratio and Proportion Ex 7D

Question 1.
If a : b = 5 : 3; find: \(\frac { 5a – 3b }{ 5a + 3b }\)
Solution:

Question 2.
If x : y = 4 : 7; find the value of (3x + 2y) : (5x + y).
Solution:

Question 3.
If a : b = 3 : 8, find the value of \(\frac { 4a + 3b }{ 6a – b }\)
Solution:

Question 4.
If (a – b): (a + b) = 1 : 11, find the ratio (5a + 4b + 15) : (5a – 4b + 3).
Solution:
(a – b) : (a + b) = 1 : 11
Let a – b = x, then a + b = 11x
Adding we get, 2a = 12x ⇒ a = 6x
Subtracting, -2b = -10x ⇒ b = 5x

Question 5.
Find the number which bears the same ratio to \(\frac { 7 }{ 33 }\) that \(\frac { 8 }{ 21 }\) does to \(\frac { 4 }{ 9 }\).
Solution:
Let x be the required number, then

Question 6.

Solution:

Question 7.
Find \(\frac { x }{ y }\) ; when x² + 6y² = 5xy.
Solution:

Question 8.
If the ratio between 8 and 11 is the same as the ratio of 2x – y to x + 2y, find the value of \(\frac { 7x }{ 9y }\)
Solution:

Question 9.
Divide ₹ 290 into A, B and C such that A is \(\frac { 2 }{ 5 }\) of B and B : C = 4 : 3.
Solution:
Total amount = ₹ 1290
A = \(\frac { 2 }{ 5 }\) B and B : C = 4 : 3
⇒ A : B = 2 : 5 and B : C = 4 : 3
LCM of 5, 4 = 20
A : B = 2 x 4 : 5 x 4 = 8 : 20
and B : C = 4 x 5 : 3 x 5 = 20 : 15
A : B : C = 8 : 20 : 15
Sum of ratios = 8 + 20 + 15 = 43

Question 10.
A school has 630 students. The ratio of the number of boys to the number of girls is 3 : 2. This ratio changes to 7 : 5 after the admission of 90 new students. Find the number of newly admitted boys.
Solution:
Number of students = 630
Ratio in boys and girls = 3 : 2

Question 11.
What quantity must be subtracted from each term of the ratio 9 : 17, to make it equal to 1 : 3?
Solution:
Let x be subtracted from each term such that
\(\frac { 9 – x }{ 17 – x }\) = \(\frac { 1 }{ 3 }\)
⇒ 17 – x = 27 – 3x
⇒ -x + 3x = 21 – 17
⇒ 2x = 10
⇒ x = 5

Question 12.
The monthly pocket money of Ravi and Sanjeev are in the ratio 5 : 7. Their expenditures are in the ratio 3 : 5. If each save Rs. 80 every month, find their monthly pocket money. [2012]
Solution:
Let the monthly pocket money of Ravi and Sanjeev be 5x and 7x respectively.
Also, let their expenditure be 3y and 5y respectively. So,
5x – 3y = 80 …(i)
and 7x – 5y = 80 …(ii)
Multiplying (i) by 7 and (ii) by 5 and subtracting, we get
4y = 160 ⇒ y = 40
From (i), 5x = 80 + 3 x 40 = 200 ⇒ x = 40
So, monthly pocket money of Ravi = Rs. 5 x 40 = Rs. 200
and monthly pocket money of Sanjeev = Rs. 7 x 40 = Rs. 280

Question 13.
The work done by (x – 2) men in (4x + 1) days and the work done by (4x + 1) men in (2x – 3) days are in the ratio of 3 : 8. Find the value of x.
Solution:
(x – 2) men can do a work in = (4x +1) days
1 man can do a work in = (4x +1) (x-2) days ….(i)
Again (4x + 1) men can do work in = 2x – 3 days
1 man can do the work in = (2x – 3) (4x + 1) days ….(ii)
From (i) and (ii), we get
(4x + 1) (x – 2) : (2x – 3) (4x + 1) = 3 : 8
⇒ (4x + 1) (x – 2) x 8 = (2x – 3) (4x + 1) x 3
⇒ 8 (4x² – 8x + x – 2) – 3 (8x² + 2x – 12x – 3)
⇒ 32x² – 64x + 8x – 16 = 24x² + 6x – 36x – 9
⇒ 32x² – 64x + 8x – 24x² – 6x + 36x – 16 + 9 = 0
⇒ 8x² – 70x + 44x – 7 = 0
⇒ 8x² – 26x – 7 = 0
⇒ 8x² – 28x + 2x – 7 = 0
⇒ 4x ( 2x – 7) + 1 (2x – 7) = 0
⇒ (2x – 7) (4x + 1) = 0
Either 2x – 7 = 0, then x = \(\frac { 7 }{ 2 }\)
or 4x + 1 = 0, then x = \(\frac { -1 }{ 4 }\) but it is not possible.
x = \(\frac { 7 }{ 2 }\) or 3.5 Ans.

Question 14.
The bus fare between two cities is increased in the ratio 7 : 9. Find the increase in the fare, if :
(i) the original fare is Rs. 245 ;
(ii) the increased fare is Rs. 207.
Solution:
The increase in bus fare between two cities is in the ratio = 7 : 9.
(i) If the original fare is Rs. 245
then increase fare = Rs. 245 x \(\frac { 9 }{ 7 }\) = Rs. 315
Increase = Rs. 315 – Rs. 245 = Rs. 70
(ii) The increased fare is Rs. 207
Original fare = Rs. \(\frac { 207 x 7 }{ 9 }\) = Rs. 161
Increase = Rs. 207 – Rs. 161 = Rs. 46

Question 15.
By increasing the cost of entry ticket to a fair in the ratio 10 : 13; the number of visitors to the fair has decreased in the ratio 6 : 5. In what ratio has the total collection increased or decreased ?
Solution:
Increase in the entry tickets = 10 : 13.
But decrease in visitors = 6 : 5
Let original price of per ticket = Rs. 10
Then increased in price will be = Rs. 13
Collection in first case = Rs. 10 x 6 = Rs. 60
and collection in second case = Rs. 13 x 5 = Rs. 65
Hence increase in collection will be = Rs. 60 : Rs. 65 = 12 : 13

Question 16.
In a basket, the ratio between the number of oranges and the number of apples is 7 : 13. If 8 oranges and 11 apples are eaten, the ratio between the number of oranges and the number of apples becomes 1 : 2. Find the original number of oranges and the original number of apples in the basket.
Solution:
The ratio in number of oranges and apples = 7 : 13
Let number of oranges = 7x
Then number of apples = 13x
When 8 oranges and 11 apples are eaten, then
According to the sum,

Original number of oranges = 7x = 7 x 5 = 35
and number of apples = 13x = 13 x 5 = 65

Question 17.
In a mixture of 126 kg of milk and water, milk and water are in ratio 5 : 2. How much water must be added to the mixture to make this ratio 3 : 2?
Solution:
Mixture of milk and water = 126 kg
Ratio in milk and water = 5 : 2
Quantity of milk = \(\frac { 126 }{ 5 + 2 }\) x 5

Question 18.
(a) If A : B = 3 : 4 and B : C = 6 : 7, find:
(i) A : B : C
(ii) A : C
(b) If A : B = 2 : 5 and A : C = 3 : 4, find: A : B : C
Solution:


A : B : C = 6 : 15 : 8

Question 19.
(i) If 3A = 4B = 6C ; find A : B : C.
(ii) If 2a = 3b and 4b = 5c, find a : c
Solution:

Question 20.
Find the compound ratio of:
(i) 2 : 3, 9 : 14 and 14 : 27.
(ii) 2a : 3b, mn : x² and x : n.
(iii) √2 : 1, 3 : √5 and √20 = 9.
Solution:
(i) Compound ratio of 2 : 3, 9 : 14 and 14 : 27

Question 21.
Find the duplicate ratio of:
(i) 3 : 4
(ii) 3√3 : 2√5
Solution:

Question 22.
Find triplicate ratio of:
(i) 1 : 3
(ii) \(\frac { m }{ 2 }\) : \(\frac { n }{ 3 }\)
Solution:

Question 23.
Find sub-duplicate ratio of:
(i) 9 : 16
(ii) (x – y)⁴ : (x + y)⁶
Solution:

Question 24.
Find sub-triplicate ratio of:
(i) 64 : 27
(ii) x³ : 125y³
Solution:

Question 25.
Find the reciprocal ratio of :
(i) 5 : 8
(ii) \(\frac { x }{ 3 }\) : \(\frac { y }{ 7 }\)
Solution:

Question 26.
If (x + 3) : (4x + 1) is the duplicate ratio of 3 : 5, find the value of x.
Solution:
(x + 3) : (4x + 1) is the duplicate ratio of 3 : 5

Question 27.
If m : n is the duplicate ratio of m + x : n + x; show that x² = mn.
Solution:

Question 28.
If (3x – 9) : (5x + 4) is the triplicate ratio of 3 : 4, find the value of x.
Solution:
(3x – 9) : (5x + 4) is the triplicate ratio of 3 : 4

⇒ 27 (5x + 4) = 64 (3x – 9)
⇒ 135x + 108 = 192x – 576
⇒ 192x – 135x = 108 + 576
⇒ 57x = 684
⇒ x = 12

Question 29.
Find the ratio compounded of the reciprocal ratio of 15 : 28, the sub-duplicate ratio of 36 : 49 and the triplicate ratio of 5 : 4.
Solution:
Reciprocal ratio of 15 : 28

Question 30.
(a) If r² = pq, show that p : q is the duplicate ratio of (p + r) : (q + r).
(b) If (p – x) : (q – x) be the duplicate ratio of p : q then show that : \(\frac { 1 }{ p }\) + \(\frac { 1 }{ q }\) = \(\frac { 1 }{ x }\)
Solution:

Hope given Selina Concise Mathematics Class 10 ICSE Solutions Chapter 7 Ratio and Proportion Ex 7A are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.

Selina Concise Mathematics Class 10 ICSE Solutions Chapter 11 Geometric Progression Ex 11C

These Solutions are part of Selina Concise Mathematics Class 10 ICSE Solutions. Here we have given Selina Concise Mathematics Class 10 ICSE Solutions Chapter 11 Geometric Progression Ex 11C

Other Exercises

• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 11 Geometric Progression Ex 11A
• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 11 Geometric Progression Ex 11B
• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 11 Geometric Progression Ex 11C
• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 11 Geometric Progression Ex 11D
• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 11 Geometric Progression Additional Questions

Question 1.
Find the seventh term from the end of the series :
√2, 2, 2√2,……32
Solution:
√2, 2, 2√2,……32
Here a = √2
r = \(\frac { 2 }{ \surd 2 } =\surd 2\)
and l =32

Question 2.
Find the third term from the end of the GP.
\(\frac { 2 }{ 27 } ,\frac { 2 }{ 9 } ,\frac { 2 }{ 3 } ,….162\)
Solution:
G.P is \(\frac { 2 }{ 27 } ,\frac { 2 }{ 9 } ,\frac { 2 }{ 3 } ,….162\)
a = \(\\ \frac { 2 }{ 27 } \)
r = \(\frac { 2 }{ 9 } \div \frac { 2 }{ 27 } \)
= \(\frac { 2 }{ 9 } \times \frac { 27 }{ 2 } \)
= 3
l = 162

Question 3.
For the G.P. \(\frac { 1 }{ 27 } ,\frac { 1 }{ 9 } ,\frac { 1 }{ 3 } …..81\)
find the product of fourth term from the beginning and the fourth term from the end.
Solution:
\(\frac { 1 }{ 27 } ,\frac { 1 }{ 9 } ,\frac { 1 }{ 3 } …..81\)
a = \(\\ \frac { 2 }{ 27 } \)
r = \(\frac { 1 }{ 9 } \div \frac { 1 }{ 27 } \)
= \(\frac { 1 }{ 9 } \times \frac { 27 }{ 1 } \)
= 3

Question 4.
If for a G.P., pth, qth and rth terms are a, b and c respectively ;
prove that :
{q – r) log a + (r – p) log b + (p – q) log c = 0
Solution:
In a G.P
T_p = a,
T_q = b,
T_r = c

Question 5.
If a, b and c in G.P., prove that : log aⁿ, log bⁿ and log cⁿ are in A.P.
Solution:
a, b, c are in G.P.
Let A and R be the first term and common ratio respectively.
Therefore,
a = A
b = AR
c = AR²
log a = log A
log b = log AR = log A + log R
log c = log AR² = log A + 2log R
log a, log b and log c are in A.P.
If 2log b = log a + log c
If 2[logA + logR] = log A + log A + 2log R
If 2log A + 2log R = 2log A + 2log R
which is true.
Hence log a, log b and log c are in A.P.

Question 6.
If each term of a G.P. is raised to the power x, show that the resulting sequence is also a G.P.
Solution:
Let a, b, c are in G.P.
Then b² = ac …(i)
Now a^x, b^x + c^x will be in G.P. if (b^x)² = a^x.c^x
=> (b^x)² = a^x.c^x
=>(b²)^x = (ac)^x
Hence a^x, b^x, c^x are in G.P. (∴ b² = ac)
Hence proved.

Question 7.
If a, b and c are in A.P. a, x, b are in G.P. whereas b, y and c are also in G.P. Show that : x², b², y² are in A.P.
Solution:
2 b = a + c _(i)
a, x, b are in G.P.
x² = ab _(ii)
and b, y, c in G.P.
y² = bc _(iii)
Now x² + y² = ab + bc
= b(a + c)
= b x 2b [from(i)]
= 2 b²
Hence x², b², y² are in G.P.

Question 8.
If a, b, c are in G.P. and a, x, b, y, c are in A.P., prove that :
(i)\(\frac { 1 }{ x } +\frac { 1 }{ y } =\frac { 2 }{ b } \)
(ii)\(\frac { a }{ x } +\frac { c }{ y } =2\)
Solution:
a, b, c are in G.P.
b² = ac
a, x, b, y, c are in A.P.
2x = a + b and 2y = b + c

Question 9.
If a, b and c are in A.P. and also in G.P., show that: a = b = c.
Solution:
a, b, c are in A.R
2 b = a + c ….(i)
Again, a, b, c are in G.P.

Hope given Selina Concise Mathematics Class 10 ICSE Solutions Chapter 11 Geometric Progression Ex 11C are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.

Selina Concise Mathematics Class 10 ICSE Solutions Chapter 6 Solving Problems (Based on Quadratic Equations) Ex 6E

These Solutions are part of Selina Concise Mathematics Class 10 ICSE Solutions. Here we have given Selina Concise Mathematics Class 10 ICSE Solutions Chapter 6 Solving Problems Ex 6E.

Other Exercises

• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 6 Solving Problems Ex 6A
• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 6 Solving Problems Ex 6B
• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 6 Solving Problems Ex 6C
• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 6 Solving Problems Ex 6D
• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 6 Solving Problems Ex 6E

Question 1.
The distance by road between two towns A and B is 216 km., and by rail it is 208 km. A car travels at a speed of x km/hr. and the train travels at a speed which is 16 km/hr faster than the car. Calculate:
(i) the time taken by the car to reach town B from A, in terms of x ;
(ii) the time taken by the train, to reach town B from A, in terms of x.
(iii) If the train takes 2 hours less than the car, to reach town B, obtain an equation in x, and solve it.
(iv) Hence, find the speed of the train. [1998]
Solution:
Distance between two stations by road = 216 km and by rail = 208 km.
Speed of car = x km/hr.
and speed of train = (x + 16) km/ hr.

⇒ 8x + 3456 = 2×2 + 32x
⇒ 2x² + 32x – 8x – 3456 = 0
⇒ 2x² + 24x – 3456 = 0
⇒ x² + 12x – 1728 = 0 (Dividing by 2)
⇒ x² + 48x – 36x – 1728 = 0
⇒ x (x + 48) – 36 (x + 48) = 0
⇒ (x + 48 ) (x – 36) = 0
Either x + 48 =0, then x = – 48 which is not possible.
or x – 36 = 0, then x = 36
(iv) Speed of train = x + 16 = 36 + 16 = 52 km/hr.

Question 2.
A trader buys x articles for a total cost of Rs. 600.
(i) Write down the cost of one article in terms of x. If the cost per article were Rs. 5 more, the number of articles that can be bought for Rs. 600 would be four less.
(ii) Write down the equation in x for the above situation and solve it for x. [1999]
Solution:
C.P. of x articles = Rs. 600

⇒ 600x = (x – 4) (600 + 5x) (By cross multiplication)
⇒ 600x = 600x + 5x² – 2400 – 20x
⇒ 5x² – 20x – 2400 = 0
(ii) x² – 4x – 480 = 0
⇒ x² – 24x + 20x – 480 = 0
⇒ x (x – 24) + 20 (x – 24) = 0
⇒ (x – 24) (x + 20) = 0 (Zero Product Rule)
Either x – 24 = 0, then x = 24
or x + 20 = 0, then x = -20 Which is not possible.
Hence no. of articles = 24

Question 3.
A hotel bill for a number of people for overnight stay is Rs. 4,800. If there were 4 people more, the bill each person had to pay would have reduced by Rs. 200. Find the number of people staying overnight. [2000]
Solution:
Amount of the bill = Rs. 4800
Let the number of persons staying overnight = x
Then amount to be paid by each person


⇒ x (x + 12) – 8 (x + 12) = 0
⇒ (x + 12) (x – 8) = 0 (Zero Product Rule)
Either x + 12 = 0, then x = -12 which is not possible being negative
or x – 8 = 0, then x = 8
Hence no. of persons staying overnight = 8

Question 4.
An aeroplane travelled a distance of 400 km at an average speed of x km/hr. On the return journey, the speed was increased by 40 km/hr. Write down an expression lor the time taken for :
(i) the omvard journey;
(ii) the return journey.
If the return journey took 30 minutes less than the on ward journey, write down an equation in x and find its value. |2002]
Solution:
Distance between A and B = 400 km.
Speed of aeroplane onward journey = x km/hr.
and Speed of aeroplane on return journey = (x + 40) km/hr.
Now time taken for onward journey = \(\frac { 400 }{ x }\) hrs.


Which is not possible being negative
or x – 160 = 0, then x = 160
x = 160

Question 5.
Rs. 6,500 were divided equally among a certain number of persons. Had there been 15 persons more, each would have got Rs. 30 less. Find the original number of persons.
Solution:
Let original number of persons = x
Amount = Rs. 6,500

⇒ 30x² + 450x – 97500 = 0 (Dividing by 30)
⇒ x² + 15x – 3250 = 0
⇒ x² + 65x – 50x – 3250 = 0
⇒ x (x + 65) – 50 (x + 65) = 0
⇒ (x + 65) (x – 50) = 0
Either x + 65 = 0, then x = -65 which is not possible.
or x – 50 = 0, then x = 50
Original number of persons = 50

Question 6.
A plane left 30 minutes later than the scheduled time and in order to reach its destination 1500 km. away in time, it has to increase its speed by 250 km./hr. from its usual speed. Find its usual speed.
Solution:
Let the usual speed of plane = x km/hr.
Distance = 1500 km.
Increased speed = (x + 250) km./hr.
Now, according to the condition,

⇒ x² + 250x – 750000 = 0
⇒ x² + 1000x – 750x – 750000 = 0
⇒ x (x + 1000) – 750 (x + 1000) = 0
⇒ (x + 1000) (x – 750) = 0
Either x + 1000 = 0, then x = – 1000 But it is not possible.
or x – 750 = 0, then x = 750
Usual speed of plane = 750 km/hr

Question 7.
Two trains leave a railway station at the same time. The first train travels due west and the second train due north. The first train travels 5 km/hr faster than the second train. If after 2 hours, they are 50 km apart, find the average speed of each train.
Solution:
Let the speed of first train = x km/hr.
Then speed of second train = (x – 5) km/ hr.
In 2 hours, first train will travel = 2x km.
and second train will travel = 2 (x – 5) km.
According to the condition,
2x + 2 (x – 5) = 50
⇒ 2x + 2x – 10 = 50
⇒ 4x = 50 + 10 = 60
x = 15 km/hr.
and speed of second train = 15 – 5 = 10 km/hr.

Question 8.
The sum S of first n even natural numbers is given by the relation S = n (n + 1). Find n if the sum is 420.
Solution:
S = n (n + 1) and x = 420
⇒ n (n + 1) = 420
⇒ n² + n – 420 = 0
⇒ n² + 21n – 20n – 420 = 0
⇒ n (n + 21) – 20 (n + 21) = 0
⇒ (n + 21) (n – 20) = 0
Either n + 21 =0, then n = -21 which is not possible as it is negative
or n – 20 = 0 then n = 20

Question 9.
The Sum of the ages of a father and his son is 45 years. Five year ago, the product of their ages (in years) was 124. Determine their present ages.
Solution:
Let age of father = x years
Then age of his son = (45 – x) years (sum = 45 years)
5 years ago,
The age of father = (x – 5) years
and age of son = 45 – x – 5 = (40 – x) years
According to the condition,
(x – 5) (40 – x) = 124
⇒ 40x – x² – 200 + 5x = 124
⇒ -x² + 45x – 200 – 124 = 0
⇒ -x² + 45x – 324 = 0
⇒ x² – 45x + 324 = 0
⇒ x² – 36x – 9x + 324 = 0
⇒ x (x – 36) – 9 (x – 36) = 0
⇒ (x – 36) (x – 9) = 0
Either x – 36 = 0, then x = 36
or x – 9 = 0, then x = 9, but it is not possible as age of father cannot be less than his son.
Age of father = 36 years
and age of son = 45 – 36 = 9 years

Question 10.
In an auditorium, seats were arranged in rows and columns. The number of rows w as equal to the number of seats in each row. When the number of rows was doubled and the number of seats in each row was reduced by 10, the total number of seats increased by 300. Find :
(i) the number of rows in the original arrangement.
(ii) the number of seats in the auditorium after re-arrangement. [2003]
Solution:
Let the number of rows in the auditorium = x
No. of seats in each row = x
and no. of total seats in the auditorium =
x x x = x²
In second case,
No. of rows = 2x
and no. of seats in each row = x – 10
Then the total seats will = x² + 300
Now, according to the condition,
2x (x – 10) = x² + 300
⇒ 2x² – 20x = x² + 300
⇒ 2x² – x² – 20x – 300 = 0
⇒ x² – 30x + 10x – 300 = 0
⇒ x (x – 30) + 10 (x – 30) = 0
⇒ (x – 30) (x + 10) = 0 (Zero Product Rule)
Either x – 30 = 0, then x = 30
or x + 10 = 0, then x = -10 Which not possible.
(i) No. of rows in original arrangement = 30
(ii) and no. of seats after re-arrangements = x² + 300 = (30)2 + 300 = 900 + 300 = 1200

Question 11.
Mohan takes 16 days less than Manoj to do a piece of work. If both working together can do it in 15 days, how many days will Mohan alone complete the work?
Solution:
Let time taken by Mohan = x days
Time taken by Manoj = (x + 16) days

⇒ 30x + 240 = x² + 16x
⇒ x² + 16x – 30x – 240 = 0
⇒ x² – 14x – 240 = 0
⇒ x² – 24x + 10x – 240 = 0
⇒ x (x – 24) + 10 (x – 24) = 0
⇒ (x + 10) (x – 24) = 0
Either x + 10 = 0, then .x = -10 But it is not possible
or x – 24 = 0, then x = 24
Mohan can do the work in = 24 days

Question 12.
Two years ago. a man’s age was three times the square of his son’s age. In three years time, his age will be four times his son’s age. Find their present ages.
Solution:
2 years ago,
let son’s age = x
Man’s age = 3x
Son’s present age = x + 2
and man’s age = 3x² + 2
and 3 years after,
Son’s age = x + 2 + 3 = x + 5
Man’s age 3x² + 2 + 3 = 3x² + 5
According to condition,
3x² + 5 = 4 (x + 5)
⇒ 3x² + 5 = 4x + 20
⇒ 3x² – 4x + 5 – 20 = 0
⇒ 3x² – 4x – 15 = 0
⇒ 3x² – 9x + 5x – 15 = 0
⇒ 3x (x – 3) + 5 (x – 3) = 0
⇒ (x – 3) (3x + 5) = 0
Either x – 3 = 0, then x = 3
or 3x + 5 = 0, then 3x = -5 ⇒ x = \(\frac { -5 }{ 3 }\)
But it is not possible.
x = 3
Son’s present age = x + 2 = 3 + 2 = 5 years
and man’s present age = 3x² + 2 = 3(3)² + 2 = 27 + 2 = 29 years

Question 13.
In a certain positive fraction, the denominator is greater than the numerator by 3. If 1 is subtracted from the numerator and the denominator both. the fraction reduces by \(\frac { 1 }{ 14 }\) Find the fraction.
Solution:
In first case.
Let numerator of a fraction = x
then, its denominator = x + 3

Question 14.
In a two digit number, the ten’s digit is bigger. The product of the digits is 27 and the difference between two digits is 6. Find the number.
Solution:
Difference of digits = 6
Let one’s digit = x
Then ten’s digit = x + 6
and number = x + 10 (x + 6) = x + 10x + 60 = 11x + 60
But product of digits = 27
x (x + 6) = 27
⇒ x² + 6x – 27 = 0
⇒ x² + 9x – 3x – 27 = 0
⇒ x (x + 9) – 3 (x + 9) = 0
⇒ (x + 9) (x – 3) = 0
Either x + 9 = 0, then x = – 9 But it is not possible
or x – 3 = 0, then x = 3
Number = 11x + 60 = 11 x 3 + 60 = 33 + 60 = 93

Question 15.
Some school children on an excursion by a bus to a picnic spot at a distance of 300 km. While returning, it was raining and the bus had to reduce its speed by 5 km/ hr and it took two hours longer for returning. Find the time taken to return.
Solution:
Distance = 300 km.
Let speed of the bus = x km/hr.

⇒ x² – 5x = 750
⇒ x² – 5x – 750 = 0
⇒ x² – 30x + 25x – 750 = 0
⇒ x (x – 30) + 25 (x – 30) = 0
⇒ (x – 30) (x + 25) = 0
Either x – 30 = 0, then x = 30
or x + 25 = 0, then x = -25, but it is not possible as it is negative
Speed of the bus = 30 km/hr
and time taken while returning = \(\frac { 300 }{ x }\) = \(\frac { 300 }{ 25 }\) = 12 hours

Question 16.
Rs. 480 is divided equally among ‘x’ children. If the number of children were 20 more then each would have got Rs. 12 less. Find ‘x’.
Solution:
Total amount = Rs. 480
Number of children = x

⇒ x² + 20x – 800 = 0
⇒ x² + 40x – 20x – 800 – 0
⇒ x (x + 40) – 20 (x + 40) = 0
⇒ (x + 40) (x – 20) = 0
Either x + 40 = 0, then x = – 40 which is not possible being negative
or x – 20 = 0 then x = 20
Number of children = 20

Question 17.
A bus covers a distance of 240 km at a uniform speed. Due to heavy rain its speed gets reduced by 10 km/h and as such it takes two hrs longer to covers the total distance. Assuming the uniform speed to be ‘x’ km/h, form an equation and solve it to evaluate ‘x’ (2016)
Solution:
Let the original speed be x km/hr
Time taken by the bus with moving at speed x km/h = \(\frac { 240 }{ x }\)
Time taken by the bus with moving at speed (x – 10) km/h = \(\frac { 240 }{ x – 10 }\)
According to the given condition,

⇒ x (x – 10) = 10 x 120
⇒ x² – 10x = 1200
⇒ x² – 10x – 1200 = 0
⇒ x² – 40x + 30x – 1200 = 0
⇒ x (x – 40) + 30 (x – 40) = 0
⇒ (x – 40) (x + 30) = 0
⇒ x – 40 = 0 or x + 30 = 0
⇒ x = 40 or x = -30
Since, the speed cannot be negative, the uniform speed is 40 km/h

Hope given Selina Concise Mathematics Class 10 ICSE Solutions Chapter 6 Solving Problems Ex 6E are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.

Selina Concise Mathematics Class 10 ICSE Solutions Chapter 24 Measures of Central Tendency (Mean, Median, Quartiles and Mode) Ex 24E

These Solutions are part of Selina Concise Mathematics Class 10 ICSE Solutions. Here we have given Chapter 24 Measures of Central Tendency (Mean, Median, Quartiles and Mode) Ex 24E.

Other Exercises

• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 24 Measures of Central Tendency Ex 24A
• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 24 Measures of Central Tendency Ex 24B
• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 24 Measures of Central Tendency Ex 24C
• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 24 Measures of Central Tendency Ex 24D
• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 24 Measures of Central Tendency Ex 24E

Question 1.
The following distribution represents the height of 160 students of a school

Draw an ogive for the given distribution taking 2 cm = 5 cm of height on one axis and 2 cm = 20 students on the other axis. Using the graph, determine :
(i) The median height.
(ii) The inter quartile range.
(iii) The number of students whose height is above 172 cm
Solution:
The cumulative frequency table may be prepared as follows :


Now, we take height along x-axis and number of students along the y-axis. Now, plot the point (145, 12), (150, 32), (155, 62), (160, 100), (165, 124), (170, 140), (175, 152) and (180, 160). On the graph paper and join them with free hand.
(i) Here N = 160 ⇒ \(\frac { N }{ 2 }\) = 80
Which is even now take a point A on the y-axis representing 80. Through A draw horizontal line meeting the ogive at B. From B, draw BC ⊥ x-axis, meeting the x-axis at C.
The abscissa of C is 157.5 So, median = 157.5 cm
(ii) Proceeding in the same way as we have done in above, we have, Q₁ = 152 and Q₃ = 164
So, inter quartile range = Q₃ – Q₁ = 164 – 152 = 12 cm
(iii) From the ogive, we see that the number of students whose height is less than 172 is 145.
No. of students whose height is above 172 cm = 160 – 145 = 15

Question 2.
The following table gives the weekly wages of workers in a factory.

Calculate : (i) the mean, (ii) the modal class, (iii) the number of workers getting weekly w ages below Rs. 80 and (iv) the number of workers get¬ting Rs 65 or more but less than Rs. 85 as weekly wages. [2002]
Solution:

(ii) Modal class 55-60 (It has maximum frequency)
(iii) No. of workers getting wages below Rs. 80 = 60
(iv) No. of worker getting Rs. 65 is more but less than 85 as weekly wages = 37

Question 3.
Draw an ogive for the data given below and from the graph determine :
(i) the median marks,
(ii) the number of students who obtained more than 75% marks ?



Through 60.5 th marks, draw a line segment parallel to x-axis which meets the curve at A.
From A, draw a line segment perpendicular to, x-axis meeting at B.
∴ B is the median = 43 (approx.)
No. of students who obtained upto 75% marks in the test =111
∴ No. of students who obtained more than 75% = 120- 111 =9

Question 4.
The mean of 1, 7, 5, 3, 4, and 4 is m. The numbers 3,2,4,2,3,3 and p have mean m-1 and median q. Find p and q.
Solution:
Mean of 1,7, 5, 3,4, and 4 = \(\frac { 24 }{ 6 }\) =4
∴ m = 4.
Now mean of 3,2,4,2,3,3 and p = m- 1= 4- 1 = 3
i.e. 17+p = 3xn when n =7
17 + p = 3×7 = 21
⇒ p = 21 – 17 = 4
Median of 3, 2,4,2, 3, 3 and 3 is q
Arranging in ascending order, 2,2, 3,3,3,3,4,4
Mean = 4th terms is 3.
∴ q = 3

P.Q.
The marks of 200 students in a test were recorded as follows :

Construct the cumulative frequency table. Draw an ogive and use it to find :
(i) the median and
(ii) the number of students who score more than 35 % marks.
Solution:

Through 100 th scores, draw a line segment parallel to x-axis which meets the curve at A. From A, draw a line segment perpendicular to it which meets at B.
∴ Median = 52.5
No. of students who score more than 35% marks.
= 200 – 28 = 172

Question 5.
In a malaria epidemic, the number of cases diagnosed were as follows :
Date (July) 1 2 3 4 5 6 7 8 9 10 11 12 Number 5 12 20 27 46 30 31 18 11 5 0 1 On what days does the mode, the upper and lower quartiles occur ?
Solution:

(i) Mode = 5th July (because it has the maximum frequencies i,e. 46)

Question 6.
The incomes of the parents of 100 students in a class in a certain university are tabulated below :

(i) Draw a cumulative frequency curve to estimate the median income.
(ii) If 15 % of the students ae given freeships on the basis of the income of their parents, find the annual income of parents, below which the freeships will be awarded.
(iii) Calculate the Arithmetic mean.
Solution:
(Cummulative Frequancy table)

(i) No. of terms= 100
∴ Mean = \(\frac { 100 }{ 2 }\) = 50th term
Through 50 mark, draw a line segment parallel to x-axis which meets the curve at A. From A, draw a perpendicular to x-axis meeting it at B, B is the median.
∴ B = 17.6 thousands
(ii) Upper quartile = 100 x \(\frac { 3 }{ 4 }\) = 75th term
From the Curve Q₃ = 23.2
(iii) Lower-quartile = 100 x \(\frac { 1 }{ 4 }\)=25th term
From the curve Q₁ = 12.8
∴ Inter-quartile range = Q₃– Q₁ = 23.2 – 12.8
= 10.4 thousands
(iv) 15% of 100 students = \(\frac { 100 x 15 }{ 100 }\) = 15
From C.F. 15, draw a horizontal line which intersects the curve at P. From P, draw a perpendicular to x-axis meeting it at 11.2
∴ Freeship to parents = Rs. 11.2 thousands upto
the income of Rs. 11.2 thousands

Question 7.
The marks of 20 students in a test were as follows : 2, 6, 8, 9, 10, 11, 11, 12, 13, 13, 14, 14, 15, 15, 15, 16, 16, 18, 19 and 20. Calculate-
(i) the mean
(ii) the median
(iii) the mode. [2002]
Solution:
Arranging in ascending order,
2, 6, 8, 9, 10, 11, 11, 12, 13, 13, 14, 14, 15, 15, 15, 15, 16, 16, 18, 19, 20
No. of terms = 20
Ix = 2 + 6 + 8 + 9+ 10+ 11 + 11 + 12+ 13 + 13 + 14 + 14 + 14 + 15 + 15 + 15 + 16 + 16 + 18 +
19 + 20 = 257

(iii) Mode = 15 (as it has maximum frequency i.e. it has 3)

Question 8.
The marks obtained by 120 students in a Mathematics test are given below:

Draw an ogive for the given distribution on a graph sheet. Use a suitable scale for your ogive. Use your ogive to estimate :
(i) the median
(ii) the number of students who obtained more than 75% marks in a test ?
(iii) the number of students who did not pass in the test if the pass percentage was 40. [2002]
(iv) the lower quartile
Solution:


Lower Quartile (Q₁)
∴ \(\frac { N }{ 4 }\)  = \(\frac { 120 }{ 4 }\)  = 30
From a point B (30) on v-axis, draw a line parallel to x- axis meeting the curve at Q and from Q Draw a line parallel to .Y-axis meeting it at 30.
∴ Lower quartile = 30
Through 60.5th marks, draw a line segment parallel to x-axis which meets the curve at A. From A, draw a line segment perpendicular to, x-axis meeting at B.
∴ B is the median = 43 (approx.)
No. of students who obtained upto 75% marks in the test = 110
∴ No. of students who obtained more than 75% = 120- 110 = 10
No. of students who obtained less than 40% marks in the test = 52 (∴ in the graph x = 40, y = 52)

P.Q.
Find the mean for the following frequency distribution: [2003]

Solution:

P.Q.
Draw a histogram and hence estimate the mode for the following frequency distribution: [2003]

Solution:
(i) Draw the histogram.

(ii) In the highest rectangle which represents the modal class draw two lines points AC and BD intersecting at P.
(iii) From P, draw a perpendicular to x-axis meeting at Q.
(iv) value of Q is the mode which is = 23

P.Q.
For die following set of data find the median :
10, 75, 3, 81, 17, 27, 4, 48, 12, 47, 9 and 15.
Solution:

P.Q.
For the following frequency distribution draw a histogram. Hence, calculate the mode.

Solution:
Histogram :
(i) Draw a histogram and make the upper corner of the rectangle
(ii) With maximum frequency A and B. Also upper corners of the two other rectangles as C and D which are the next and to maximum rectangle.

(iii) Join AD and BC which intersect at P.
(iv) From P, draw PM ⊥ X – axis
OM = 13
Hence mode = 13

Question 9.
Using a graph paper, draw an Ogive for the following distribution which shows a record of the weight in kilograms of 200 students.

Use your Ogive to estimate the following :
(i) The percentage of students weighing 55 kg or more,
(ii) The weight above which the heaviest 30% of the students fall.
(iii) The number of students who are (a) under-weight and (b) over-weight, if 55.70 kg is considered as standard weight. (2005)
Solution:

Plot the points (45. 5), (50, 22), (55, 44). (60, 89), (65, 140), (70, 171), (75, 191) and (80, 200) on the graph and join them in free hand to get an ogive as shown.

(i) From the graph, number of students weighing 55 kg or more = 200 – 44 = 156

∴ Heaviest 60 students in weight = 9 + 20 + 31 = 60 (From the graph, the required weight is 65 kg or more but less than 80 kg)
(iii) Total number of students who are (i) under weight = 47 and (ii) over weight = 152 (∴ Standard weight is 55.70 kg)

P.Q.
Using step deviation method, find the mean of the following distribution.

Solution:

P.Q.
The daily wages of 80 workers in a building project are given below :

Using graph paper, draw an Ogive for the above distribution.
Use your Ogive to estimate : (i)the median wages of the workers.
(ii) the percentage of workers who earn more than Rs. 75 day.
(iii) the upper quartile wage of the workers.
(iv) the lower quartile wage of the workers.
(v) Inter quartile range.
Solution:


Now plot the points (40, 6), (50, 16), (60, 31), (70, 50), (80, 62), (90, 70) (100, 76). and (110, 80) on the graph and join them with free hand to get an ogive as shown.
(i) Median : \(\frac { N }{ 2 }\) = \(\frac { 80 }{ 2 }\) = 40
From 40 on y-axis, draw a line parallel to x- axis meeting the curve at P. From P, draw PL ⊥ x-axis
Then L is the median which is 65
∴ Median = Rs. 65
(ii) No. of workers earning more than Rs. 75 per day
From 75 on v-axis, draw a perpendicular meeting the curved at Q and from Q( draw a line parallel to x-axis which meet y-axis at B which is 57
∴No. of workers getting more than Rs. 75 per day = 80 – 57 = 23

From 60 on y-axis, draw- a line parallel to x- axis which meets the curve at R. From R, draw a perpendicular on x-axis meeting it at N.

From 20 on y-axis, draw a line parallel to x- axis meeting the curve at S. From S, draw a perpendicular on x-axis meeting it at T.
T is the lower quartile (Q₁) which is 53.5
∴ Q₁ = Rs. 53.50
(v) Inter quartile range = Q₃ – Q₁ = Rs. 78-53.50 = Rs. 24.50

Question 10.
The distribution given below shows the marks obtained by 25 students in an aptitude test. Find the mean, median and mode of the distribution.

Solution:

Mode = Marks with maximum frequency is 6 ∴ Mode = 6

Question 11.
The mean of the following distribution is 52 and the frequency of class interval 30-40 is Find ‘f’ .Find ‘ f ‘.

Solution:

Question 12.
The monthly income of a group of 320 employees in a company is given below:

Draw an ogive of the given distribution on a graph sheet taking 2 cm = Rs. 1000 on one axis and 2 cm= 50 employees on the other axis.
From the graph determine
(i)the median wage
(ii)the number of employees whose income is below Rs. 8500.
(iii)If the salary of a senior employee is above Rs. 11,500, find the number of senior employees in the company.
(iv) the upper quartile.
Solution:


(i)For median wage, Take OP =\(\frac { 320 }{ 2 }\) =160 on y-axis, Draw a line PQ || x-axis and from Q,
draw QM ⊥ x-axis, abcissa of M point is 9400 ⇒ Median = Rs. 9400
(ii) Take OM’ = 8500 on.t-axis. Draw Q’M’|| toy-axis and P’Q’ || X-axis
Where ordinate of P’ is 92.5
There are approximately 93 employees whose monthly wage is below Rs. 8500
(iii) There are approximately 18 employees whose salary is above Rs. 11500.
(iv) Upper quartile
Mark a point A ony-axis on \(\frac { 3N }{ 4 }\) = \(\frac { 3 x 320 }{ 4 }\)= 240 and draw a line AB || X-axis, then draw BB’
⊥ x-axis abscissa of B’ is upper quartile i.e., Rs. 10250.

Question 13.
A Mathematics aptitude test of 50 students was recorded as follows :

Draw a histogram for the above data using a graph paper and locate the mode.
Solution:

1. Draw the histogram with given data.
2. Inside the highest rectangle which represents the maximum frequency (or modal class), draw two lines AC and BD diagonally from the upper comer C and D or adjacent rectangle which intersect at K.
3. Draw KL ⊥ X-axis.
Value ofL is the mode which is 82.5 (approx).

Question 14.
Marks obtained by 200 students in an examination are given below :

Draw an ogive for the given distribution taking 2 cm = 10 marks on one axis and 2 cm = 20 students on the other axis. Using the graph, determine
(i) The median marks.
(ii) The number of students who failed if minimum marks required to pass is 40.
(iii) If scoring 85 and more marks is considered as grade one, find the number of students who secured grade one in the examination.
Solution:


(i) Median is 57.
(ii)44 students failed.
(iii) No. of students who secured grade one = 200 – 188 = 12

Question 15.
Marks obtained by 40 students in a short assessment are given below, where a and ft are two missing data.

If the mean of the distribution is 7.2, find a and b.
Solution:

Question 16.
Find the mode and median of the following frequency distribution :

Solution:

Question 17.
The median of the following observations 11, 12, 14, (x – 2), (x + 4), (x + 9), 32, 38, 47 arranged in ascending order is 24. Find the value of x and hence find the mean.
Solution:

Question 18.
The numbers 6, 8, 10, 12, 13 and x are arranged in an ascending order. If the mean of the observations is equal to the median, find the value of x. (2014)
Solution:

Question 19.
(Use a graph paper for this question.) The daily pocket expenses of 200 students in a school are given below:

Draw a histogram representing the above distribution and estimate the mode from the graph. (2014)
Solution:
Steps of construction :
(i) Draw a line BC = 6.5 cm.
(ii) Centre B and C draw arcs AB = 5.5 cm and AC = 5 cm
(iii) Join AB and AC, ABC is the required triangle,
(iv) Draw the angle bisetors of B and C. Let these bisectors meet at O.
(v) Taking O as centre. Draw a incircle which touches all the sides of the ∆ ABC.
(vi) From O draw a perpendicular to side BC which cut at N.
(vii) Measure ON which is required radius of the incircle.
ON = 1.5 cm

Question 20.
The marks obtained by 100 students in a Mathematics test are given below :

Draw an ogive for the given distribution on a graph sheet.
Use a scale of 2 cm 10 units on both axes).
Use the ogive to estimate the:
(i) median.
(ii) lower quartile.
(iii) number of students who obtained more than 85% marks in the test.
(iv) number of students failed, If the pass percentage was 35. (2014)
Solution:


N= 100
Median = \(\frac { 100 }{ 2 }\) = 50th term Median = 45
(ii) Lower quartile : (Q1)
N = 100
⇒ \(\frac { N }{ 4 }\) = \(\frac { 100 }{ 4 }\) = 25
∴ Q1 = 32
(iii) Mo. of students with 85% less = 94
∴ More than 85% marks = 100 94 6
(iv) Number of students who did not pass = 30

Question 21.
The marks obtained by 30 students in a eiass assessment of 5 marks is given below :

Calculate the mean, median and mode of the above distribution. (2015)
Solution:


Which is between 10 and 20
∴ Median = 3
(ii) Mode frequency of 3 is the greatest
∴ Mode = 3

Question 22.
The weight of 50 workers is given below :

Draw an ogive of the given distribution using a graph sheet. Take 2 cm = 10 kg on one axis and 2 cm = 5 workers along the other axis. Use the ogive drawn to estimate the following:
(i) the upper and lower quartiles.
(ii) if weighing 95 Kg and above is considered overweight, find the number of workers who are overweight. (2015)
Solution:
Plot the points (60, 4), (70, 11), (80, 22), (90, 36), (100, 42) (110, 47) and (120, 50) on the graph and join them in order with free hand.
This is the required ogive


(i) Upper Quartile = \(\frac { 50 x 3 }{ 4 }\) th term = \(\frac { 150 }{ 4 }\) th = -37.5th term
Lower Quartile = \(\frac { 50 }{ 4 }\) th = 12.5th term
Upper quartile is 42 kg and lower quartile is 72 kg.
(ii) 95 kg and above are over weight
∴ No. of over weight students are 50 – 39 = 11 students.

Question 23.
The mean of following number is 68. Find the value of ‘x’. 45, 52, 60, x, 69, 70, 26, 81 and 94. Hence estimate the median. (2016)
Solution:

Question 24.
The table shows the distribution of the scores obtained by 160 shooters in a shooting competition. Use a graph sheet and draw an ogive for the distribution. (Take 2 cm = 10 scores on the X-axis and 2 cm = 20 shooters on the Y-axis). (2016)

Use your graph to estimate the following :
(i) The median.
(ii) The interquartile range.
(iii) The number of shooters who obtained a score of more than 85%.
Solution:


Through mark 80 on y-axis, draw a horizontal line which meets the ogive drawn at point Q.
Through Q, draw a vertical line which meets the x-axis at the mark of 43(app.).
∴ Median = 43
(ii) Since the number of terms = 160


(iii) Since 85% scores = 85% of 100 = 85
Through mark for 85 on x-axis, draw a vertical line which meets the ogive drawn at point B.
Through the point B, draw a horizontal line which meets thej-axis at the mark of 148 = 160- 148= 12
So, the number of shooters who obtained more than 85% score is 12.

Question 25.
The histogram below represents the scores obtained by 25 students in a Mathematics mental test. Use the data to :
(i) Frame a frequency distribution table
(ii) To calculate mean
(iii) To determine the Modal class

Solution:

(iii) Here the maximum class frequency is 8, and the class corresponding to this frequency is 20-30. So, the modal class is 20-30.

Hope given Selina Concise Mathematics Class 10 ICSE Solutions Chapter 24 Measures of Central Tendency Ex 24E  are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.

Selina Concise Mathematics Class 10 ICSE Solutions Chapter 11 Geometric Progression Ex 11B

These Solutions are part of Selina Concise Mathematics Class 10 ICSE Solutions. Here we have given Selina Concise Mathematics Class 10 ICSE Solutions Chapter 11 Geometric Progression Ex 11B

Other Exercises

• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 11 Geometric Progression Ex 11A
• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 11 Geometric Progression Ex 11B
• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 11 Geometric Progression Ex 11C
• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 11 Geometric Progression Ex 11D
• Selina Concise Mathematics Class 10 ICSE Solutions Chapter 11 Geometric Progression Additional Questions

Question 1.
Which term of the G.P. :
– 10, \(\frac { 5 }{ \surd 3 } ,-\frac { 5 }{ 6 } ,….-\frac { 5 }{ 72 } ? \)
Solution:
– 10, \(\frac { 5 }{ \surd 3 } ,-\frac { 5 }{ 6 } ,….\)
Here a = – 10
r = \(\frac { 5 }{ \surd 3 } \div \left( -10 \right) \)

n – 1 = 4
=> n = 4 + 1 = 5
It is 5th term

Question 2.
The fifth term of a G.P. is 81 and its second term is 24. Find the geometric progression.
Solution:
In a G.P.
T₅ = ar⁴ = 81
T₂ = ar = 24

Question 3.
Fourth and seventh terms of a G.P. \(\\ \frac { 1 }{ 18 } \) are \(– \frac { 1 }{ 486 } \) respectively. Find the GP.
Solution:
In a G.P.

Question 4.
If the first and the third terms of a G.P. are 2 and 8 respectively, find its second term
Solution:
In a G.P.
T₁ = 2, and T₃ = 8
=>a = 2 and ar² = 8
Dividing, we get
r² = \(\\ \frac { 8 }{ 2 } \) = 4 = (2)²
r = 2
Second term = ar = 2 x 2 = 4

Question 5.
The product of 3rd and 8th terms of a G.P. is 243. If its 4th term is 3, find its 7th term.
Solution:
Let a be first term and r be common ratio, then
T₃ = ar²
T₈ = ar⁷
T₃ x T₈ = ar² x ar⁷
= 243

Question 6.
Find the geometric progression with 4th term = 54 and 7th term = 1458.
Solution:
In a G.P.
T₄ = 54 and T₇ = 1458
Let a be the first term and r be the common
ratio, then

Question 7.
Second term of a geometric progression is 6 and its fifth term is 9 times of its third term. Find the geometric progression. Consider that each term of the G.P. is positive.
Solution:
In a G.P.
T₂ = 6,
T₅ = 9 x T₃
Let a be the first term and r be the common ratio

Question 8.
The fourth term, the seventh term and the last term of a geometric progression are 10, 80 and 2560 respectively. Find its first term, common ratio and number of terms.
Solution:
In a G.P.
T₄= 10,
T₇ = 80 and l = 2560
Let a be the first term and r be the common ratio. Therefore

Question 9.
If the 4th and 9th terms of a G.P. are 54 and 13122 respectively, find the GP. Also, find its general term.
Solution:
In a G.P.
T₄ = 54 and T₉ = 13122
Let a be the first term and r be the common ratio

Question 10.
The fifth, eight and eleventh terms of a geometric progression are p, q and r respectively. Show that : q² = pr.
Solution:
In a G.P.
T₅ = p,
T₈ = q and T₁₁ = r
To show that q² = pr
Let a be the first term and r be the common ratio, therefore
ar⁴ = p, ar⁷ = q and ar¹⁰ = r
Squaring the ar⁷ = q

Hope given Selina Concise Mathematics Class 10 ICSE Solutions Chapter 11 Geometric Progression Ex 11B are helpful to complete your math homework.

If you have any doubts, please comment below. Learn Insta try to provide online math tutoring for you.